Nicomachus’ Theorem

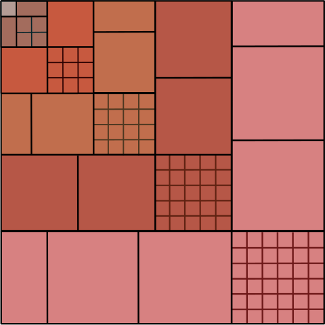
http://commons.wikimedia.org/wiki/File:%D7%9E%D7%A9%D7%A4%D7%98_%D7%A0%D7%99%D7%A7%D7%95%D7%9E%D7%90%D7%9B%D7%95%D7%A1.svg
Image: Wikimedia Commons

In 100 C.E., Nicomachus of Gerasa observed that

13 + 23 + 33 + … + n3 = (1 + 2 + 3 + … + n)2

Or “the sum of the cubes of 1 to n is the same as the square of their sum.” The diagram above demonstrates this neatly: Counting the individual squares shows that

1 × 12 + 2 × 22 + 3 × 32 + 4 × 42 + 5 × 52 + 6 × 62
= 13 + 23 + 33 + 43 + 53 + 63
= (1 + 2 + 3 + 4 + 5 + 6)2

| Science & Math