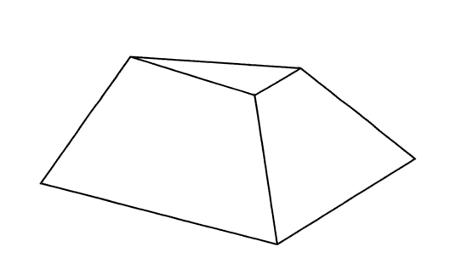
Here’s a subtly impossible figure devised by UC-Santa Cruz computer scientist David Huffman. If it’s a three-sided pyramid, then its edges define the intersections of three planes and should meet in a single point. But they don’t:

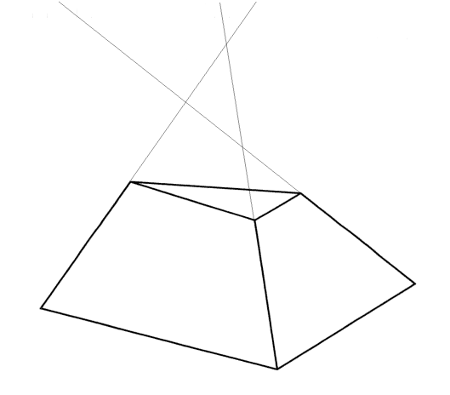
This is intriguing because the figure doesn’t immediately look impossible. In Vagueness and Contradiction, philosopher Roy Sorensen writes, “The impossibility of an appearance is sometimes concealed without overloading our critical capacities.”

Possibly this is because we sense that other solutions are possible that can reconcile the error. Zenon Kulpa points out that the pyramid becomes intelligible if we imagine that the farther side hides a fourth edge, giving the figure four sides rather than three. He describes two families of such solutions in “Are Impossible Figures Possible?”, Signal Processing, May 1983.