
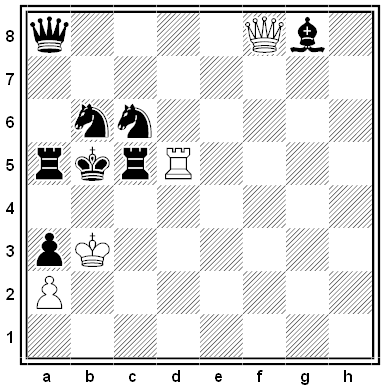
1. By A.F. Rockwell. White to mate in two moves. (Solutions are below.)
2. In 1866 Sam Loyd asked: Suppose you’re playing White against an opponent who’s required to mirror every move you make — if you play 1. Nf3 he must play 1. … Nf6, and so on. Can you design a game in which your eighth move forces your opponent to checkmate you with a nonmirror move?
3. An endgame study by J.A. Miles. “White to play and draw the game.”
|
SelectClick for Answer |
1. Qc5! and the queen or bishop will mate. (From A Collection of Chess Problems, 1890.)
2. Loyd’s solution is 1. e4 e5 2. Ke2 Ke7 3. Ke3 Ke6 4. Qf3 Qf6 5. Ne2 Ne7 6. b3 b6 7. Ba3 Ba6:
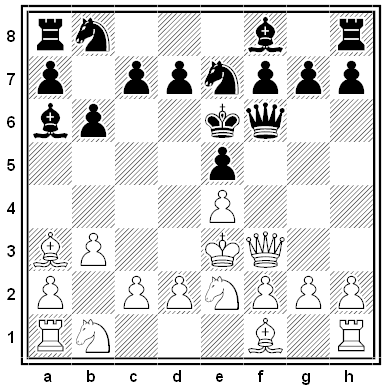
Now 8. Nd4+ forces 8. … exd4, mating White. (From Alain C. White, Sam Loyd and His Chess Problems, 1962.)
3. This is the frontispiece to the collection Chess Stars: A Galaxy of Self-Mates, from 1888. Unfortunately no solution is given!
White is already nearly stalemated — if he can get rid of his queen he’ll have his draw. But the only line I can find is the ridiculous and ungainly 1. Qf1+ Nc4 2. Qxc4+ Kb6 3. Qa6+ Kc7 4. Qc8+ Kb6 5. Qb7+, where Black must take the queen. All Black’s moves are forced if he wants to avoid a draw, but this is such an unbeautiful line that I feel sure it can’t be the intended solution. Any ideas?
|



