A problem from the 2004 Harvard-MIT Math Tournament:
Zach chooses five numbers from the set {1, 2, 3, 4, 5, 6, 7} and tells their product to Claudia. She finds that this is not enough information to tell whether the sum of Zach’s numbers is even or odd. What is the product that Zach tells Claudia?
|
SelectClick for Answer |
420. Giving the product of the five chosen numbers is equivalent to giving the product of the two unchosen numbers. The only possible products that are produced by more than more than one pair of numbers are 12 (produced by {3, 4} and {2, 6}) and 6 (produced by {1, 6} and {2, 3}). In the second case, the sum of both pairs is odd, so the sum of the five chosen numbers would have to be odd too. So the first must be the case, and product of Zach’s five chosen numbers is
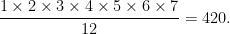
(From Titu Andreescu et al., 104 Number Theory Problems, 2007.)
|
