Here’s another interesting source of complementary sequences. Take any positive irrational number, say , and call it X. Call its reciprocal Y; in this case , or about 0.7. Add 1 to each of X and Y and we get

1 + X ≈ 2.4

1 + Y ≈ 1.7.

Now make a table of the approximate multiples of 1 + X and 1 + Y:

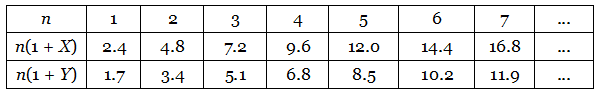
If we drop the fractional part of each number in the table, we’re left with two complementary sequences — every number 1, 2, 3, … appears in one sequence or the other, but never in both.

They’re called Beatty sequences, after Sam Beatty of the University of Toronto, who discovered them in 1926. A pretty proof by A. Ostrowski and J. Hyslop appears in the March 1927 issue of the American Mathematical Monthly and in Ross Honsberger’s Ingenuity in Mathematics (1970).