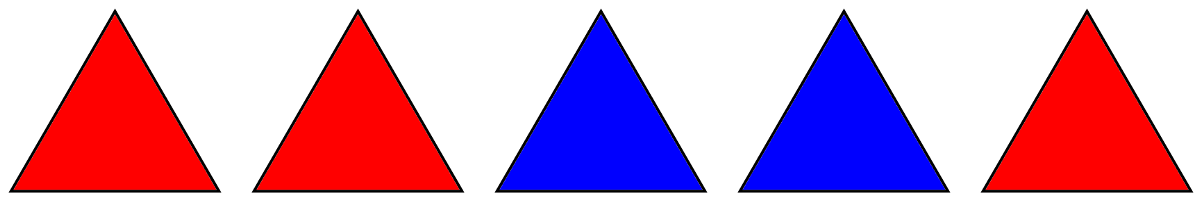
A puzzle by MIT mathematician Tanya Khovanova:
Three logicians walk into a bar. Each is wearing a hat that’s either red or blue. Each logician knows that the hats were drawn from a set of three red and two blue hats; she doesn’t know the color of her own hat but can see those of her companions.
The waiter asks, “Do you know the color of your own hat?”
The first logician answers, “I do not know.”
The second logician answers, “I do not know.”
The third logician answers, “Yes.”
What is the color of the third logician’s hat?
|
SelectClick for Answer |
If Logician #1 saw that both of her companions were wearing blue hats, then she’d know that her own hat must be red. But she says, “I do not know.” That tells us that either #2 or #3 (or both) is wearing a red hat.
Logician #2 understands this. So if she saw that #3 was wearing a blue hat, then she’d know that she herself was wearing a red one. But she too says, “I do not know.” That means that Logician #3 must be wearing a red hat.
Logician #3, realizing this, says so.
Interestingly, Khovanova says her puzzle was inspired by a joke:
Three logicians walk into a bar. The waitress asks, “Do you all want beer?”
The first logician answers, “I do not know.”
The second logician answers, “I do not know.”
The third logician answers, “Yes.”
She suggests that it might be valuable to find a way to convert jokes into puzzles and vice versa.
(Tanya Khovanova, “Hat Puzzles,” Recreational Mathematics Magazine 1:2 [September 2014], 5-10.)
|

