The Sea Island Problem - Futility Closet

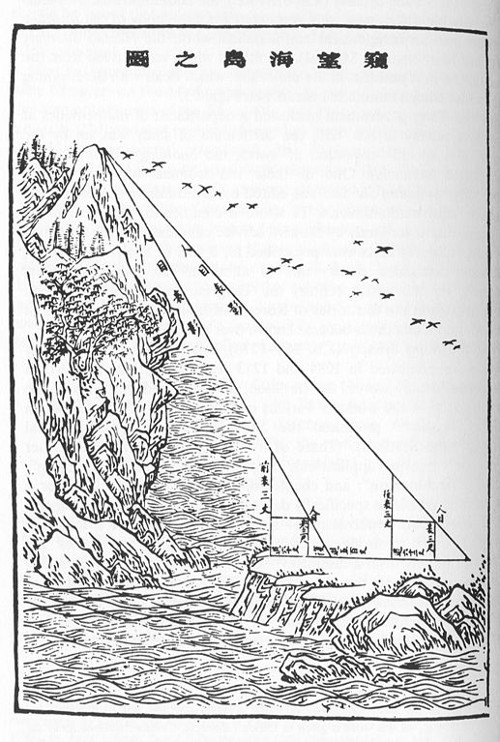
The Chinese mathematician Liu Hui offered this technique in a text composed about 500 years after Euclid. We’re on the mainland, and we want to find the height of a mountain on a distant island without crossing the sea.

Liu Hui showed that this can be accomplished by setting up two poles of a known height in a line with the mountain …

https://commons.wikimedia.org/wiki/File:Sea_Island_Measurement.jpg — Image: Wikimedia Commons

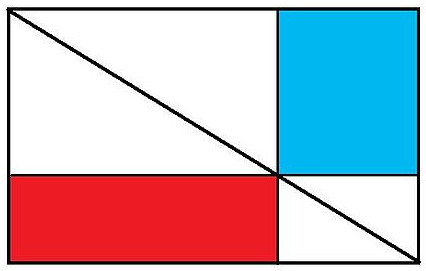
… and by appealing to a principle of complementary rectangles — here the red and the blue rectangles have the same area:

https://commons.wikimedia.org/wiki/File:Rectangle_in_triangle.jpg — Image: Wikimedia Commons

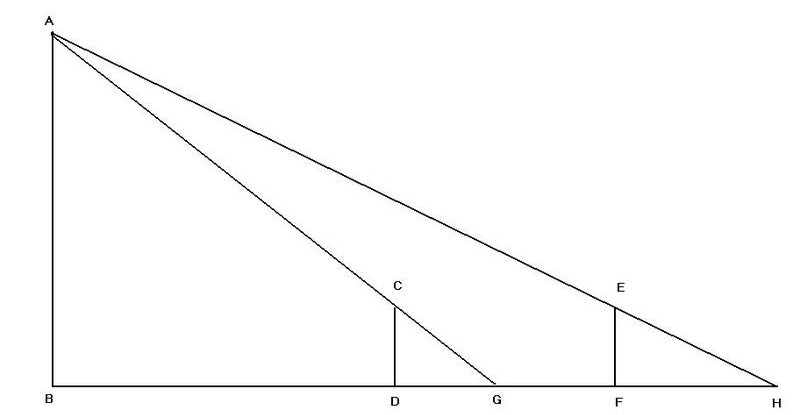
By using that principle it’s possible to recast the problem in terms of values that we can measure: the height of the poles (CD), the “offset” from which the top of the mountain can just be sighted from ground level over the top of each pole (DG and FH), and the distance between the poles (DF). Putting all that together we can find both the height of the mountain:

$\displaystyle \frac{CD \times DF}{FH - DG} + CD$

and the distance between the first pole and the mountain:

$\displaystyle \frac{DG \times DF}{FH - DG}$

without ever leaving the mainland. Penn State University mathematician Frank Swetz concluded that “in the endeavours of mathematical surveying, China’s accomplishments exceeded those realized in the West by about one thousand years.”