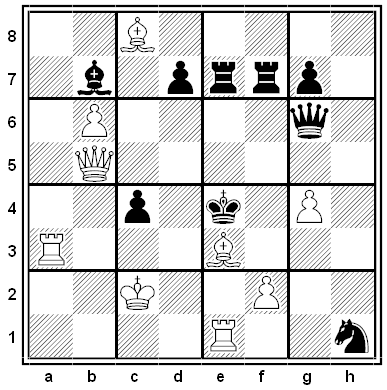
A “grid-chess” problem by E. Visserman, from Fairy Chess Review, 1954. A grid divides the board into 16 large squares, and each move by each side must cross at least one line of the grid. For example, in this position it would be illegal for the black king to move to f3. How can White mate in two moves?
|
SelectClick for Answer |
1. Ra5 threatens 2. Qxc4, which would be mate (again, the black king can’t flee to f3). Black has no defense:
1. … Qf6 2. Qf5#
1. … Qe6 2. Qe5#
1. … Qd6 2. Bxb7#
1. … Qc6 2. Qd5#
1. … Qxb6 2. Bxb6#
1. … d5 2. Bc5#
1. … Rxf2+ 2. Bxf2#
(Via John M. Rice, An ABC of Chess Problems, 1970.)
|

