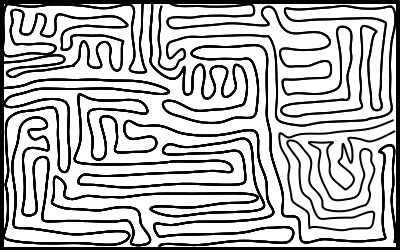
Your eccentric uncle has dropped you into the middle of Twine Island, which is festooned with one continuous loop of twine. The twine never crosses itself, but it snakes everywhere, and the island is too hilly for you to see the whole layout at once. As a character-building exercise, your uncle offers you a million dollars if you can determine whether you’re inside the loop or outside. How can you do this?
|
SelectClick for Answer |
Walk in any direction until you reach the shore, counting the number of times you cross the twine. If the final number is odd then your starting point was inside the loop; if not then you were outside.
Related: Is it possible to draw a straight line that intersects every side of a 999-sided polygon?
No. A straight line can’t intersect every side of any polygon with an odd number of sides. Each time the line crosses a side, one of the polygon’s vertices falls on one side of the line and another vertex falls on the other side. If we succeeded there would be an equal number of vertices on each side of the line, but an odd-sided polygon has an odd number of vertices.
(That last one is by Polish mathematician Paul Vaderlind.)
|

