A problem by Polish mathematician Paul Vaderlind:
Each child in a school plays either tennis or soccer. One-ninth of the tennis players also play soccer, and one-seventh of the soccer players also play tennis. Do more than half the children play tennis?
|
SelectClick for Answer |
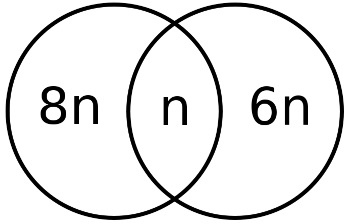
Some number of kids play both sports. Call that n. Nine times this many play tennis, and seven times this many play soccer. So it follows that 8n play tennis only, 6n play soccer only, and there are n + 8n + 6n kids altogether. The proportion of kids who play tennis is 9n to 15n, or 3/5, which is more than half.
From Vaderlind’s excellent 2002 book The Inquisitive Problem Solver, with Richard Guy and Loren Larson.
|