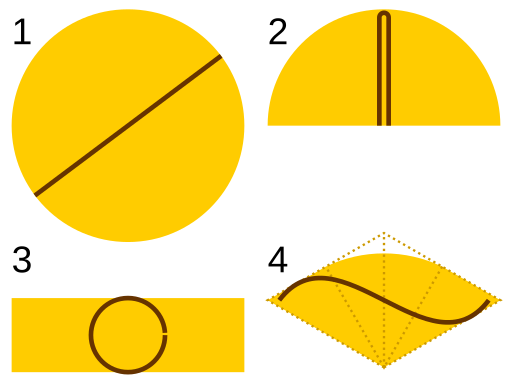
Suppose you have a publicity-seeking inchworm and want to keep him to yourself. What’s the smallest cover you can contrive to keep him hidden? He can writhe into any shape that an inch-long creature can take; you must always be able to turn your shape to keep him covered.

Strangely, we don’t yet know the answer to this question. Mathematician Leo Moser first posed it in 1966, and various proposals have driven the upper bound as low as π/12 ≈ 0.2618, but we still don’t know whether smaller covers are possible. It’s known as Moser’s worm problem.