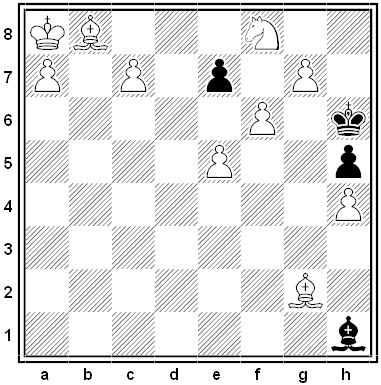
This chess problem, from Wolfgang Pauly’s 1912 Theory of Pawn Promotion, is a selfmate in two: How can White force Black to deliver checkmate within two moves, despite Black’s best effort to avoid doing so? White moves first.
|
SelectClick for Answer |
The bishops are nicely aligned with White’s king; if Black had no other option, he’d have to play … Bxg2, giving mate. But White can’t simply withdraw his bishop along the long diagonal, as then Black could shift his own bishop mildly to g2, avoiding mate.
What other options does White have? He mustn’t move his knight, as that would allow the Black king to move. 1. e6 allows Black to take the f6 pawn. Moving the f6 pawn allows Black to take the g7 pawn. Promoting the g pawn to a queen or a rook counterproductively protects the g2 bishop. Promoting it to a knight checkmates Black (!). Promoting it to a bishop almost works, but after 1. g8=B exf6 2. exf6 Bxg2+ White now has 3. Bd5, which again prevents checkmate.
The only resource that works is to promote the c7 pawn to a knight. Now Black has only two ways to avoid giving mate, and both are only temporary:
1. … exf6 2. exf6 Bxg2#
2. … e6 2. g8=B Bxg2# (as now the promoted bishop can’t reach d5)
Note that the c7 pawn must promote to a knight — any other piece could block the final check.
|

