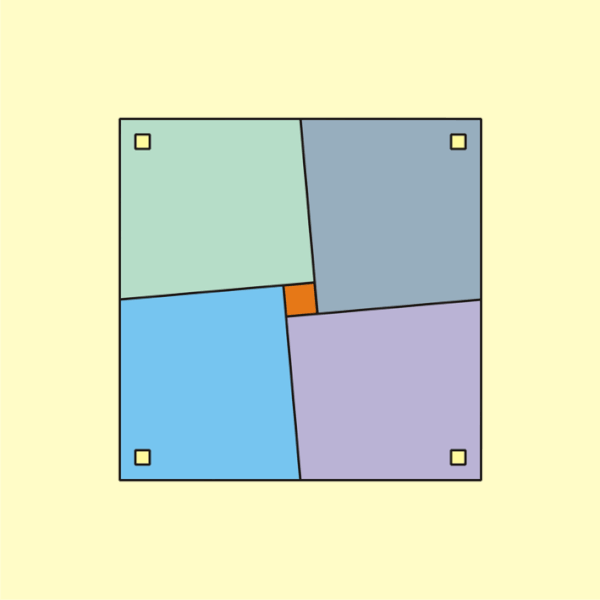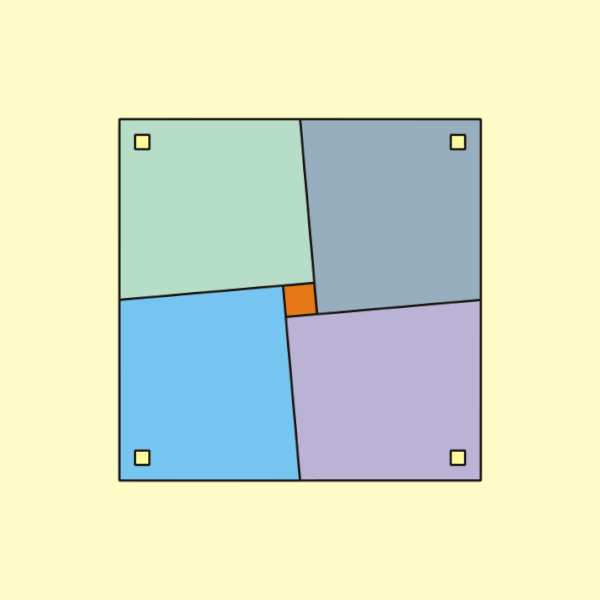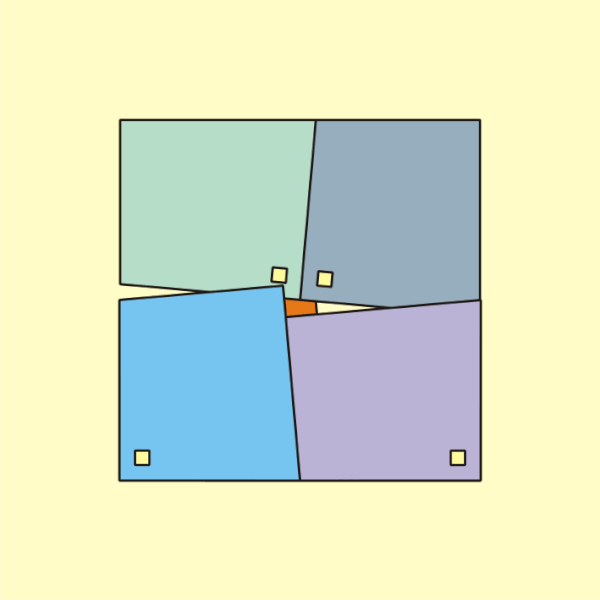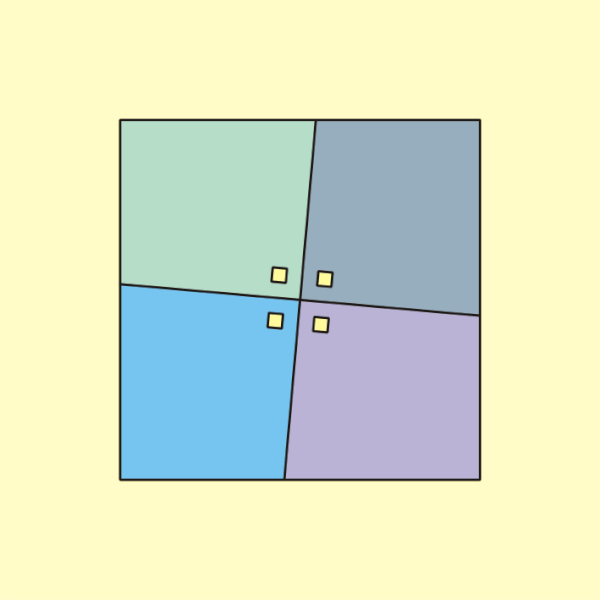
In this conundrum by Mitsunobu Matsuyama, when four congruent quadrilaterals are rotated about their centers, their area seems to increase. What has become of the red square?
The answer is that each side of the large square is slightly shorter after the rotation. If θ is the angle between two opposing sides in each quadrilateral, then the ratio of the areas of the two large squares is sec2 θ, about 0.8 percent when θ is 5°.
