One train leaves Los Angeles for New York at 60 mph.
At the same time, another train leaves New York for Los Angeles at 40 mph.
What is the distance between them one hour before they meet?
One train leaves Los Angeles for New York at 60 mph.
At the same time, another train leaves New York for Los Angeles at 40 mph.
What is the distance between them one hour before they meet?
In 1998 a retired naval pilot in California began receiving semi-coherent telephone calls from around the country blaming him for torrential rain and crop failures.
“Some of them absolutely curse me out and others just ask me, in a rather grudged way, if I can just stop the rain.”
He takes the calls with good humor and has maintained his listing in the phone book.
His name is Al Nino.
unlove
v. to cease to love
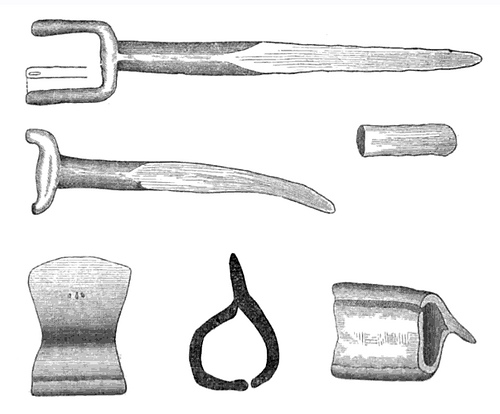
Clearing out a prehistoric mica mine in Macon County, N.C., in 1880, investigators discovered a curious collection of iron tools.
The inference to be drawn from the discovery of these iron relics, is, that some of the ‘old diggings’ are the work of Europeans, as the use of iron was unknown to the native American races. Is it not possible that there is a basis of truth in the old Cherokee tradition? That a party of Spanish explorers — and perhaps more than one — penetrated Western Carolina in search of gold, silver and other minerals, and, in some instances, finding the old mines of the Mound-builders, caused preliminary investigations of their value, does not seem improbable.
“To what expedition these Europeans belonged is a mystery. That of De Soto, according to the course traced out by Bancroft, passed within a comparatively short distance of North Carolina … From it an exploring party was sent to the north, which returned disheartened, without the precious gold, reporting the mountains impassable. Could the work have been done by stragglers from this or other parties, or have there been special expeditions to this region of which the historian has lost sight?”
(Frederic W. Simonds, “The Discovery of Iron Implements in an Ancient Mine in North Carolina,” American Naturalist, January 1881)
“Contentment is natural wealth; luxury is artificial poverty.” — Socrates

Three of the first five U.S. presidents died on Independence Day.
Already every bank of any importance probably uses calculating machines. It is not likely that the fatiguing and uncertain process of having arithmetical calculations of any sort performed in the brains of clerks will survive the improvements of which these machines are capable. Account books, invoices, and all similar documents will doubtless be written by a convenient and compendious form of combined calculating machine and typewriter, which we may suppose to be called the numeroscriptor. … It will make any kind of calculation required. Even such operations as the weighing and measurement of goods will all be done by automatic machinery, capable of recording without any possibility of error the quantity and values of goods submitted to its operation.
– T. Baron Russell, A Hundred Years Hence, 1906
A.A. Bennett offered this puzzle in the American Mathematical Monthly of May 1937:
A car with n (n > 2) passengers of different speeds of mental reaction passes through a tunnel and each passenger acquires unconsciously a smudge of soot upon his forehead. Suppose that each passenger
(1) laughs and continues to laugh as soon as and only so long as he sees a smudge upon the forehead of a fellow passenger;
(2) can see the foreheads of all his fellows;
(3) reasons correctly;
(4) will clean his own forehead when and only when his reasoning forces him to conclude that he has a smudge;
(5) knows that (1), (2), (3), and (4) hold for each of his fellows.
Show that each passenger will eventually wipe his own forehead.
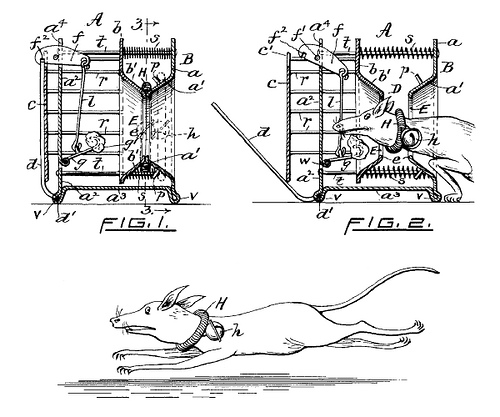
Joseph Barad and Edward Markoff were certainly humane — the rat trap they patented in 1908 merely fastened a jingling necklace around the animal’s neck and released it.
What would that accomplish? “The ‘bell-rat’ as it may be termed, then in seeking its burrow or colony announces his coming by the sounds emitted by the bells, thereby frightening the other rats and causing them to flee, thus practically exterminating them in a sure and economical manner.”
Did it work? Who knows?
New York florist Max Schling once placed an ad in the New York Times that was written entirely in shorthand.
Hundreds of curious businessmen passed the ad on to their secretaries, requesting a translation.
The secretaries read: “When getting flowers for the boss’s wife, remember Schling’s Florist.”