Semiotician Charles K. Bliss was born in Czernowitz, in Austria-Hungary, a city with a confluence of nationalities that “hated each other, mainly because they spoke and thought in different languages.” So Bliss invented a new language to encourage communication between speakers of different languages — “Blissymbols” were ideographic, meaning they conveyed ideas or concepts, and so were not beholden to any spoken language.
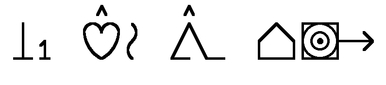
For example, the sentence above reads “I want to go to the cinema”:
- The symbol for “person” is attended by the number 1, indicating the first person.
- The heart indicates a feeling, modified by a serpentine line indicating “fire,” topped a caret, indicating that it’s a verb in this sentence.
- The symbol for “leg” also gets a caret, as it’s to be interpreted as a verb here.
- The symbol for “house” is modified by the symbol for “film,” and the arrow indicates movement.
The language never fulfilled its potential as a bridge among cultures, but it became popular in the 1970s in teaching disabled people to communicate, and an organization known as Blissymbolics Communication International oversees its applications around the world.
(Thanks, Zach.)