From the Strand, December 1901, “one of Sir John Stainer’s musical jokes, two hymns in one — in B flat or G major, according to the manner in which it is read, upside up or upside down. It was written as an autograph for a friend of his son’s.”
Author: Greg Ross
Footwork
Conclusion of a 2021 investigation by physicist Eve Armstrong of her cat’s reactions to a laser pointer:
FTake, for example, the structure that emerges in the D1-
D3-D4 slice of the four-dimensional phase space (Figure 5,
middle left). Thisf particular viewing angfffle reveals the
startfffffffffffling property offfffffffffffffffffffffffffffgrtoit
y[jlu;mj9jgffggggggggffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
ffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffffff
fffscinating and merits further investigation.
(Eve Armstrong, “My Cat Chester’s Dynamical Systems Analysyyyyy7777777777777777y7is of the Laser Pointer and the Red Dot on the Wall: Correlation, Causation, or SARS-Cov-2 Hallucination?”, arXiv preprint arXiv:2103.17058 [2021].)
“The Ingenious Patriot”
Having obtained an audience of the King an Ingenious Patriot pulled a paper from his pocket, saying:
‘May it please your Majesty, I have here a formula for constructing armor plating that no gun can pierce. If these plates are adopted in the Royal Navy our warships will be invulnerable and therefore invincible. Here, also, are reports of your Majesty’s Ministers, attesting the value of the invention. I will part with my right in it for a million tumtums.’
After examining the papers, the King put them away and promised him an order on the Lord High Treasurer of the Extortion Department for a million tumtums.
‘And here,’ said the Ingenious Patriot, pulling another paper from another pocket, ‘are the working plans of a gun that I have invented, which will pierce that armor. Your Majesty’s royal brother, the Emperor of Bang, is eager to purchase it, but loyalty to your Majesty’s throne and person constrains me to offer it first to your Majesty. The price is one million tumtums.’
Having received the promise of another check, he thrust his hand into still another pocket, remarking:
‘The price of the irresistible gun would have been much greater, your Majesty, but for the fact that its missiles can be so effectively averted by my peculiar method of treating the armor plates with a new –‘
The King signed to the Great Head Factotum to approach.
‘Search this man,’ he said, ‘and report how many pockets he has.’
‘Forty-three, Sire,’ said the Great Head Factotum, completing the scrutiny.
‘May it please your Majesty,’ cried the Ingenious Patriot, in terror, ‘one of them contains tobacco.’
‘Hold him up by the ankles and shake him,’ said the King; ‘then give him a check for forty-two million tumtums and put him to death. Let a decree issue making ingenuity a capital offence.’
— Ambrose Bierce, Fantastic Fables, 1899
Math Notes
Is every integer the sum of four perfect cubes?
Interestingly, no one knows. It’s conjectured that the answer is yes, but no one yet has been able to prove this.
Learning
“Good tests kill flawed theories; we remain alive to guess again.” — Karl Popper
“There are two possible outcomes: if the result confirms the hypothesis, then you’ve made a measurement. If the result is contrary to the hypothesis, then you’ve made a discovery.” — Enrico Fermi
“This particular thesis was addressed to me a quarter of a century ago by John Campbell, who … told me that all theories are proven wrong in time. … My answer to him was, ‘John, when people thought the Earth was flat, they were wrong. When people thought the Earth was [perfectly] spherical, they were wrong. But if you think that thinking the Earth is spherical is just as wrong as thinking the Earth is flat, then your view is wronger than both of them put together.’ The basic trouble, you see, is that people think that ‘right’ and ‘wrong’ are absolute; that everything that isn’t perfectly and completely right is totally and equally wrong. However, I don’t think that’s so. It seems to me that right and wrong are fuzzy concepts.” — Isaac Asimov, The Relativity of Wrong, 1988
The Arc of Narrative
In 2020, three researchers from UT Austin and Lancaster University examined 40,000 fictional narratives and discovered a consistent linguistic pattern. Articles and prepositions such as a and the are common at the start of a story, where they set the stage by providing information about people, places, and things. As the plot progresses, auxiliary verbs, adverbs, and pronouns become more common — words that are action-oriented and social. Near the end, “cognitive tension words” such as think, realize, and because become more common, words that reflect people trying to make sense of their world.
These patterns are consistent across novels, short stories, and amateur (“off-the-cuff”) stories. “If we want to connect with an audience, we have to appreciate what information they need, but don’t yet have,” said lead author Ryan Boyd. “At the most fundamental level, humans need a flood of ‘logic language’ at the beginning of a story to make sense of it, followed by a rising stream of ‘action’ information to convey the actual plot of the story.”
At this website you can view the graphs produced by various example narratives and even analyze your own.
(Ryan L. Boyd, Kate G. Blackburn, and James W. Pennebaker, “The Narrative Arc: Revealing Core Narrative Structures Through Text Analysis,” Science Advances 6:32 [2020], eaba2196.) (Thanks, Sharon.)
Black and White
“The Elephant; or the Force of Habit”
A tail behind, a trunk in front,
Complete the usual elephant.
The tail in front, the trunk behind,
Is what you very seldom find;
If you for specimens should hunt
With trunks behind and tails in front,
That hunt would occupy you long;
The force of habit is so strong.
— A.E. Housman
Unquote

“One of the strangest things about life is that the poor, who need the money most, are the very ones that never have it.” — Finley Peter Dunne
Plato’s Number
By Wikimedia user Cmglee, a visual proof that 33 + 43 + 53 = 63:
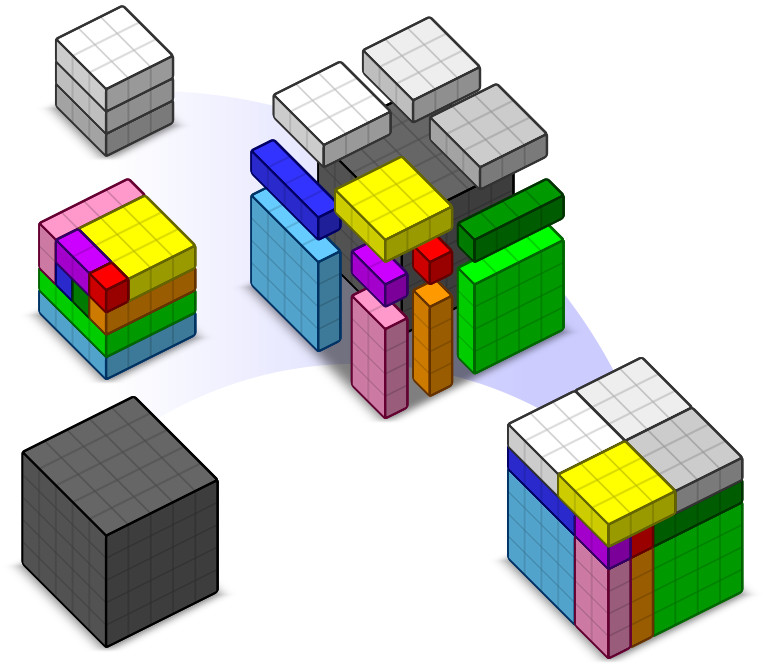
This value, 216, is sometimes called Plato’s number because it seems to correspond to this enigmatic passage in the Republic:
Now for divine begettings there is a period comprehended by a perfect number, and for mortal by the first in which augmentations dominating and dominated when they have attained to three distances and four limits of the assimilating and the dissimilating, the waxing and the waning, render all things conversable and commensurable with one another, whereof a basal four-thirds wedded to the pempad yields two harmonies at the third augmentation, the one the product of equal factors taken one hundred times, the other of equal length one way but oblong,-one dimension of a hundred numbers determined by the rational diameters of the pempad lacking one in each case, or of the irrational lacking two; the other dimension of a hundred cubes of the triad. And this entire geometrical number is determinative of this thing, of better and inferior births.
Unfortunately, Plato’s meaning is far from certain. Other values that have been proposed (with various rationales) include 1,728, 3,600, 5,040, 8,128, 17,500, 760,000, and 12,960,000. Cicero politely described the question as “obscure”; it remains open.