From Chapter 12 of Ken Follett’s novel The Pillars of the Earth:
‘My stepfather, the builder, taught me how to perform certain operations in geometry: how to divide a line exactly in half, how to draw a right angle, and how to draw one square inside another so that the smaller is half the area of the larger.’
‘What is the purpose of such skills?’ Josef interrupted.
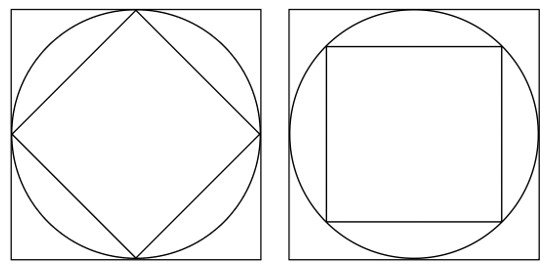
‘Those operations are essential in planning buildings,’ Jack replied pleasantly, pretending not to notice Josef’s tone. ‘Take a look at this courtyard. The area of the covered arcades around the edges is exactly the same as the open area in the middle. Most small courtyards are built like that, including the cloisters of monasteries. It’s because these proportions are most pleasing. If the middle is bigger, it looks like a marketplace, and if it’s smaller, it just looks as if there’s a hole in the roof. But to get it exactly right, the builder has to be able to draw the open part in the middle so that it’s precisely half the area of the whole thing.’
How is this done? Inscribe a diamond within a square and then rotate it 45 degrees: