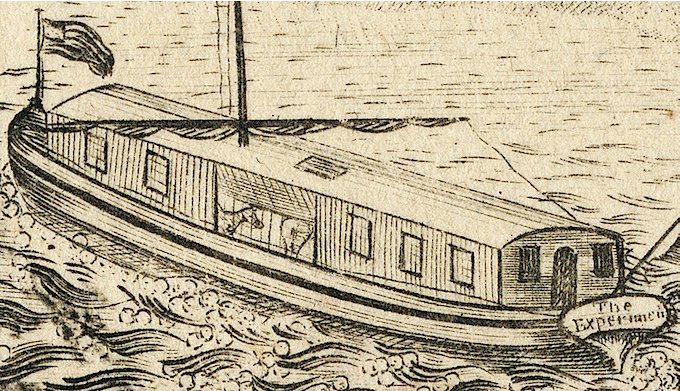
In June 1809, a ferry boat called the Experiment set out from Providence, R.I. for Pawtuxet Village. It was named for an innovative propulsion system: a screw propeller driven by eight horses on a treadmill. Unfortunately the mechanism was poorly realized — even with a favorable wind and tide, the craft made only 4 knots on her outbound journey, and on the return a gust of wind blew her into mud flats, ending her career. She was broken up and sold to remunerate the creditors, and even the patent was lost in an 1836 fire, but the innovation of the screw propeller would find a place in other designs.




