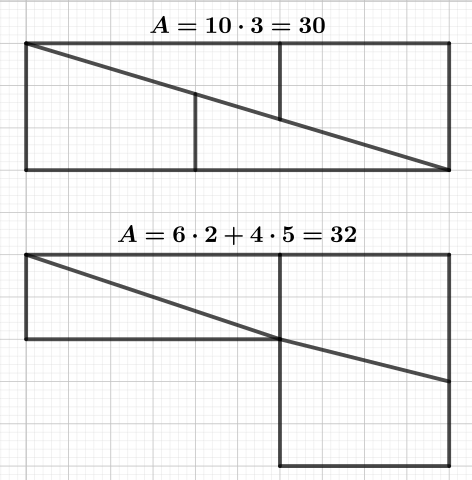
William Hooper published the oddity in 1774. The rectangle at the top measures 10 units by 3, giving an area of 30. But its dissected pieces seem to produce two other rectangles, with areas 12 and 20. Where did the two extra units come from?

William Hooper published the oddity in 1774. The rectangle at the top measures 10 units by 3, giving an area of 30. But its dissected pieces seem to produce two other rectangles, with areas 12 and 20. Where did the two extra units come from?
A variation on the grandfather paradox … is the Hitler paradox. In this one you travel back in time to murder Hitler before he starts the Second World War, thus saving millions of lives. But if you murder Hitler in, say, 1938, then the Second World War will never come about and you will have no reason to travel back in time to murder Hitler!
— J.H. Brennan, Time Travel: A New Perspective, 1997

Norman Rockwell’s image of “Rosie the Riveter,” published on the cover of the Saturday Evening Post in 1943, is based on Michelangelo’s 1509 painting Prophet Isaiah, from the Sistine Chapel ceiling.
Michelangelo’s contemporary Giorgio Vasari had written, “Anyone who studies this figure, copied so faithfully from nature, the true mother of the art of painting, will find a beautifully composed work capable of teaching in full measure all the precepts to be followed by a good painter.”
Also, Rosie is using Mein Kampf as a footrest.

In areas of mountainous terrain and wildnerness, the border between the United States and Canada is kept clear of brush and vegetation to a width of 6 meters, forming a visible line between the nations that’s visible in satellite images.
The deforested segments total more than 2,000 kilometers.
(The fourth is due to Mick Tully, the fifth to David Armstrong.)
In 1899, six years before her death at age 70, Aboriginal Tasmanian Fanny Cochrane Smith made five wax cylinder recordings of traditional Aboriginal songs and language.
They are the only recorded example of Tasmanian Aboriginal songs and the only recorded example of any Tasmanian Aboriginal language.
Emily Keene, who was present at the recording, said that when the cylinder was played back for her, Smith cried, “My poor race. What have I done.”
“We could not pacify her for a long time,” Keene said. “She thought the voice she had heard was that of her mother.”

The shortest street in York, England, is called Whip-Ma-Whop-Ma-Gate.
The name is first recorded in 1505 as Whitnourwhatnourgate, and appears later as Whitney Whatneygate.
It appears to mean “neither-one-thing-nor-the-other-street.”
In 1963, French geologist Michel Siffre descended into a glaciated cavern under the French-Italian Maritime Alps and spent more than two months without sun to “investigate time, that most inapprehensible and irreversible thing.” He could telephone scientists on the surface, who recorded the time of each call, but they never told him the time or date. From his diary:
Forty-second awakening. … I really seem to have no least idea of the passage of time. This morning, as an example, after telephoning to the surface and talking for a while, I wondered afterward how long the telephone conversation had lasted, and could not even hazard a guess. … Fifty-second awakening. … I am losing all notion of time. … When, for instance, I telephone the surface and indicate what time I think it is, thinking that only an hour has elapsed between my waking up and eating breakfast, it may well be that four or five hours have elapsed. And here is something hard to explain: the main thing, I believe, is the idea of time that I have at the very moment of telephoning. If I called an hour earlier, I would still have stated the same figure. … I am having great difficulty to recall what I have done today. It costs me a real intellectual effort to recall such things.
The outsiders could see that his waking and sleeping remained near a cycle of 24.5 hours, but Siffre’s conscious understanding of time was greatly affected. Misunderstanding the length of his day, he began to husband his rations, thinking he had weeks more to endure. At his 57th awakening, the final day of the experiment, he thought it was August 20; in fact it was September 14. “I underestimated by almost half the length of my working or waking hours; a ‘day’ that I estimated at seven hours actually lasted on the average fourteen hours and forty minutes.” NASA has pursued these inquiries to consider the implications for space travelers.
(From Jane Brox, Brilliant: The Evolution of Artificial Light, 2010.)
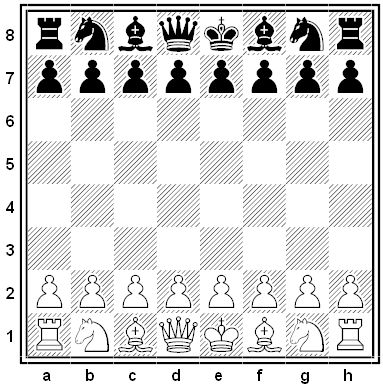
A puzzle by Henry Dudeney:
A chessboard was on the table with the pieces all set up for a game. So I asked Dr. Bates to play a game with the Major on these conditions: Whatever move Bates made throughout, with the white pieces, the Major must exactly imitate with the black, and Bates must give checkmate on the fourth move. As an experiment, Bates started off with 1. e4, and Rackford replied with 1. e5. Then Bates played 2. Qh5, and the Major had to reply with 2. Qh4. This gave me a good opportunity to explain that White cannot now play 3. QxQ, because it would be impossible for Black then to imitate the move. Neither could he play 3. Qxf7+, because Black cannot do the same thing, as he would have to get out of check. White must always make a move that Black can copy, until the checkmate is actually given on the fourth move.
“This puzzle caused great interest, and it was some time before somebody (I think it was Strangways) hit on a solution.”
The name of one chemical element appears as an unbroken string in the names of four other elements. What is the element, and what are the four?