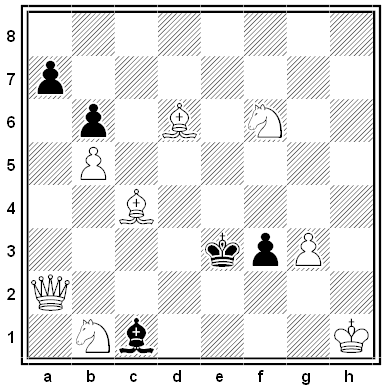
“A fairly good two-mover” from Benjamin Glover Laws’ The Two-Move Chess Problem, 1890. What’s the key move?

“A fairly good two-mover” from Benjamin Glover Laws’ The Two-Move Chess Problem, 1890. What’s the key move?

One night in 1631, pirates from the Barbary coast stole ashore at the little Irish village of Baltimore and abducted 107 people to a life of slavery in Algiers — a rare instance of African raiders seizing white slaves from the British Isles. In this week’s episode of the Futility Closet podcast we’ll describe the sack of Baltimore and the new life that awaited the captives in North Africa.
We’ll also save the Tower of London and puzzle over a controversial number.

Revenue agents in 18th-century London faced a curious challenge: how to calculate the excise tax on a barrel that was partially full of liquor. The answer was an “ullage slide rule” — this gauging rod was dipped into the barrel, some brass sliding pieces were adjusted to reflect the height of the surface, and a mathematical calculation would tell how much liquid the barrel contained.
The Science Museum says, “The calculations were very complicated.” A correspondent to the Mathematical Gazette wrote in 1990, “I well remember puzzling, unsuccessfully, over graphs and calculations of measurements until I wrote to the makers whose name was stamped on the rule and who still existed [in 1966] at the same address in London Bridge. At that time they were still making a modern equivalent for the same use by revenue officers.” More at the link below.
(Tom Martin, “Gauging: The Art Behind the Slide Rule,” Brewery History 133 [2009], 69-86.)

Ishmael narrates Moby-Dick, just as Gulliver narrates his travels and John Watson narrates the Sherlock Holmes stories. In each case we can assume that all the information presented in the literary story is imparted to us by its fictional narrator.
But the filmed version of each story contains thousands of details that are apparent to us but clearly never observed directly by the narrator. Yet it’s still the narrator who’s ostensibly telling us the story. If the narrator isn’t supplying these details, then … who is?

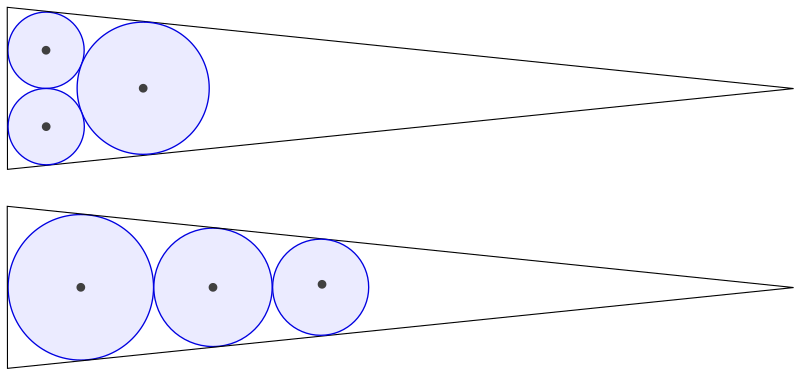
What’s the best way to squeeze three circles into a triangle so that the area of the circles is maximized? In 1803 Italian mathematician Gian Francesco Malfatti decided that the best course was to place each circle tangent to the other two and to two sides of the triangle (left) — he thought that some instance of this arrangement would give the best solution.
But that’s not actually so: In an equilateral triangle, Malfatti’s circles occupy less area than the solution on the right, found by Lob and Richmond in 1930 — their suggestion is to inscribe the largest possible circle in the triangle, then fit the second circle into one of the triangle’s three corners, and then fit the third circle into one of the five spaces now available, taking the largest available option in each case.
In the case of an equilateral triangle, Lob and Richmond’s solution is only about 1% larger than Malfatti’s. But in 1946 Howard Eves pointed out that for a long, narrow isosceles triangle (below), simply stacking three circles can cover nearly twice the area of the Malfatti circles.
Subsequent studies have borne this out — it turns out that Malfatti’s plan is never best. We now know that Lob and Richmond’s procedure will always find three area-maximizing circles — but whether their approach will work for more than three circles is an open question.
(Thanks, Larry.)
Inscriptions found in 17th-century English wedding rings, from William Jones’ Finger-Ring Lore, 1898:
A diamond ring bore the inscription “This sparke will grow.”
In 1854, a correspondent wrote to Notes and Queries asking about the origins of this couplet:
Perturbabantur Constantinopolitani
Innumerabilibus sollicitudinibus.
[“Constantinople is much perturbed.”]
He got this reply:
“When I first learned to scan verses, somewhere about thirty years ago, the lines produced by your correspondent P. were in every child’s mouth, with this story attached to them. It was said that Oxford had received from Cambridge the first line of the distich, with a challenge to produce a corresponding line consisting of two words only. To this challenge Oxford replied by sending back the second line, pointing out, at the same time, the false quantity in the word Constantinŏpolitani.”
The opening of Chapter 5 in E.M. Forster’s 1910 novel Howards End describes a concert performance of the third movement of Beethoven’s Fifth Symphony — and succinctly illustrates six ways that people listen to instrumental music:
Whether you are like Mrs. Munt, and tap surreptitiously when the tunes come — of course, not so as to disturb the others –; or like Helen, who can see heroes and shipwrecks in the music’s flood; or like Margaret, who can only see the music; or like Tibby, who is profoundly versed in counterpoint, and holds the full score open on his knee; or like their cousin, Fräulein Mosebach, who remembers all the time that Beethoven is ‘echt Deutsch’; or like Fräulein Mosebach’s young man, who can remember nothing but Fräulein Mosebach: in any case, the passion of your life becomes more vivid, and you are bound to admit that such a noise is cheap at two shillings.
“All these responses are, of course, presented in highly ironical terms,” writes University of Graz literature professor Walter Bernhart, “but surely it is a very clever brief outline of a basic typology of music reception.”
(From Werner Wolf, Walter Bernhart, and Andreas Mahler, Immersion and Distance: Aesthetic Illusion in Literature and Other Media, 2013.)
Reader Eliot Morrison, a protein biochemist, has been looking for the longest English word found in the human proteome — the full set of proteins that can be expressed by the human body. Proteins are chains composed of amino acids, and the most common 20 are represented by the letters A, C, D, E, F, G, H, I, K, L, M, N, P, Q, R, S, T, V, W, and Y. “These amino acids have different chemical properties,” Eliot writes, “and the sequence influences how the whole chain folds in three dimensions, which in turn determines the structural and functional properties of the protein.”
The longest English word he’s found is TARGETEER, at nine letters, in the uncharacterized protein C12orf42. The whole sequence of C12orf42 is:
MSTVICMKQR EEEFLLTIRP FANRMQKSPC YIPIVSSATL WDRSTPSAKH IPCYERTSVP CSRFINHMKN FSESPKFRSL HFLNFPVFPE RTQNSMACKR LLHTCQYIVP RCSVSTVSFD EESYEEFRSS PAPSSETDEA PLIFTARGET EERARGAPKQ AWNSSFLEQL VKKPNWAHSV NPVHLEAQGI HISRHTRPKG QPLSSPKKNS GSAARPSTAI GLCRRSQTPG ALQSTGPSNT ELEPEERMAV PAGAQAHPDD IQSRLLGASG NPVGKGAVAM APEMLPKHPH TPRDRRPQAD TSLHGNLAGA PLPLLAGAST HFPSKRLIKV CSSAPPRPTR RFHTVCSQAL SRPVVNAHLH
And there are more: “There are also a number of eight-letters words found: ASPARKLE (Uniprot code: Q86UW7), DATELESS (Q9ULP0-3), GALAGALA (Q86VD7), GRISETTE (Q969Y0), MISSPEAK (Q8WXH0), REELRALL (Q96FL8), RELASTER (Q8IVB5), REVERSAL (Q5TZA2), and SLAVERER (Q2TAC2).” I wonder if there’s a sentence in us somewhere.
(Thanks, Eliot.)
The difference of any pair of the numbers {1873432, 2288168, 2399057, 6560657} is a perfect square (of 644, 725, 2165, 333, 2067, or 2040).