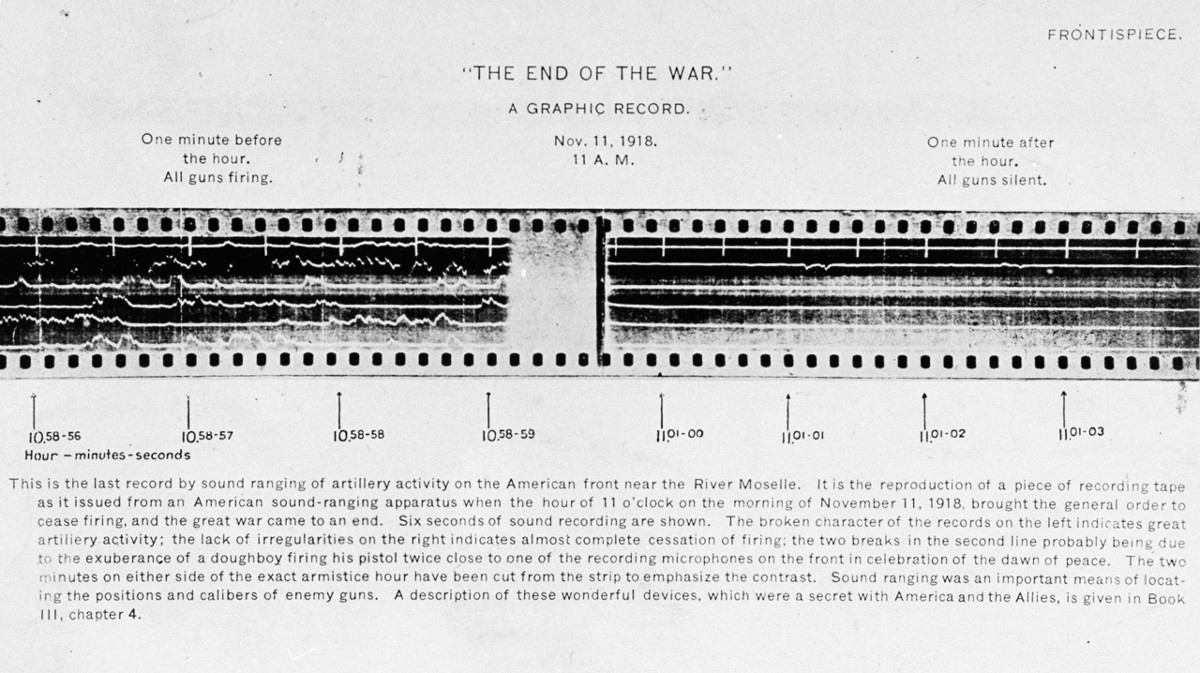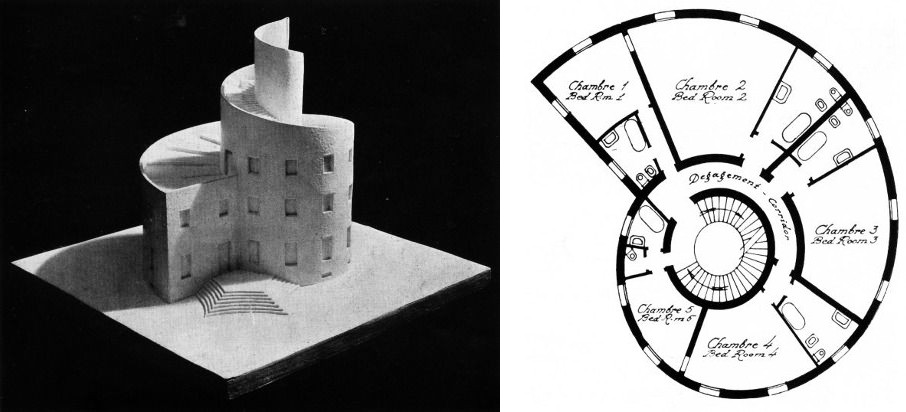
In 1930 French architect Emilio Terry offered a model of a spiral house that he called en colimaçon (“snail-style”), illustrating his view that architecture expressed a “dream to be realized.”
It looks odd, but the interior is relatively conventional. “Although the snail shape has been imposed on the exterior,” writes Ulrich Conrads in The Architecture of Fantasy, “no advantage is derived from it, either regarding the total volume or the interrelationship of the spatial sections.”