
When Saint Clare of Assisi was too ill to attend mass, an image of the service would appear on the wall of her cell.
So in 1957 Pope Pius XII designated her the patron saint of television.
(Thanks, Charlie.)

When Saint Clare of Assisi was too ill to attend mass, an image of the service would appear on the wall of her cell.
So in 1957 Pope Pius XII designated her the patron saint of television.
(Thanks, Charlie.)

In 1928 Cleveland’s Cunningham Sanitarium erected the largest hyperbaric chamber ever built, five stories tall and weighing 900 tons. It was inspired by Kansas City physician Orval J. Cunningham’s belief that diabetes and cancer are caused by anaerobic organisms that would die off if patients could be sequestered in a highly oxygenated environment.
After a year of work and a million dollars, the finished sphere was ready. It could accommodate 40 patients at a time in a climate-controlled environment of 68° and 65% humidity. With steel doors and circular portholes, it had the atmosphere of a ship. To minimize the risk of fire, none of the 36 patient rooms contained any wooden components.
Unfortunately, Cunningham ran into financial straits and had to sell the chamber after only five years. Renamed the Ohio Institute of Oxygen Therapy, it failed to attract patients, operated briefly as a general hospital, and closed finally in 1936. In 1942 the U.S. War Production Board ordered it scrapped, and the metal went into military tanks.
Peter Carey’s True History of the Kelly Gang contains no commas. (“I lost my own father at 12 yr. of age and know what it is to be raised on lies and silences my dear daughter you are presently too young to understand a word I write but this history is for you and will contain no single lie may I burn in Hell if I speak false.”)
Vanessa Place’s Dies: A Sentence is a single sentence of 120 pages.
Mathias Enard’s Zone is a single sentence of 517 pages.
Michel Thaler’s Le Train de Nulle Part contains no verbs. (“Quelle aubaine! Une place de libre, ou presque, dans ce compartiment. Une escale provisoire, pourquoi pas! Donc, ma nouvelle adresse dans ce train de nulle part: voiture 12, 3ème compartiment dans le sens de la marche. Encore une fois, pourquoi pas?”)
Christine Brooke-Rose’s Amalgamemnon is a “pragmatic lipogram” — all its verbs are conditional, future, subjunctive, etc., so that nothing is actually happening in the present: “I shall soon be quite redundant at last despite of all, as redundant as you after queue and as totally predictable, information-content zero.”
Adam Adams’ 2008 novel Unhooking a DD-Cup Bra Without Fumbling contains no Es.
My notes say that Iegor Gran’s Les Trois Vies de Lucie can be read straight through, recto pages only, or verso pages only, yielding three different stories, but I haven’t managed to find a copy to check.
It is like a play. But when the curtain falls, the one who played the king, and the one who played the beggar, and all the others — they are all quite alike, all one and the same; actors. And when in death the curtain falls on the stage of actuality … then they also are all one; they are human beings. All are that which they essentially were, something we did not see because of the difference we see. They are all human beings. The stage of art is like an enchanted world. But just suppose that some evening a common absent-mindedness confused all the actors so they thought they really were what they were representing. Would this not be, in contrast to the enchantment of art, what one might call the enchantment of an evil spirit, a bewitchment? And likewise suppose that in the enchantment of actuality (for we are, indeed, all enchanted, each one bewitched by his own distinctions) our fundamental ideas became confused so that we thought ourselves essentially to be the roles we play. Alas, but is this not the case? It seems to be forgotten that the distinctions of earthly existence are only like an actor’s costume.
— Kierkegaard, Works of Love, 1847

“I’m sure I’m not Ada, for her hair goes in such long ringlets, and mine doesn’t go in ringlets at all; and I’m sure I can’t be Mabel, for I know all sorts of things, and she, oh! she knows such a very little! Besides, she’s she, and I’m I, and — oh dear, how puzzling it all is! I’ll try if I know all the things I used to know. Let me see: four times five is twelve, and four times six is thirteen, and four times seven is — oh dear! I shall never get to twenty at that rate!”
So frets Alice outside the garden in Wonderland. Is the math only nonsense? In his book The White Knight, Alexander L. Taylor finds one way to make sense of it: In base 18, 4 times 5 actually is 12. And in base 21, 4 times 6 is 13. Continuing this pattern:
4 times 7 is 14 in base 24
4 times 8 is 15 in base 27
4 times 9 is 16 in base 30
4 times 10 is 17 in base 33
4 times 11 is 18 in base 36
4 times 12 is 19 in base 39
And here it becomes clear why Alice will never get to 20: 4 times 13 doesn’t equal 20 in base 42, as the pattern at first seems to suggest, but rather 1X, where X is whatever symbol is adopted for 10.
Did Lewis Carroll have this in mind when he contrived the story for Alice? Perhaps? In The Magic of Lewis Carroll, John Fisher writes, “It is hard … not to accept Taylor’s theory that Carroll was anxious to make the most of two worlds; the problem as interpreted by Taylor interested him, and although it wouldn’t interest Alice there was no reason why he shouldn’t use it to entertain her on the level of nonsense. It is even more difficult to suppose that he, a mathematical don, inserted the puzzle in the book without realising it.”
In the Winter 1971 edition of Jabberwocky, the quarterly publication of the Lewis Carroll Society, Taylor wrote, “If you find a watch in the Sahara Desert you don’t think it grew there. In this case we know who put it there, so if he didn’t record it that tells us something about his recording habits. It doesn’t tell us that the mathematical puzzle isn’t a mathematical puzzle.”

Giuseppe Sanmartino’s 1753 sculpture Veiled Christ is so convincing that for 200 years a legend circulated that an alchemist had transformed a real shroud into marble.
In fact a close examination shows that Sanmartino carved the whole work, shroud and all, from a single block of stone. Antonio Canova said he would give up 10 years of his own life to produce such a masterpiece.
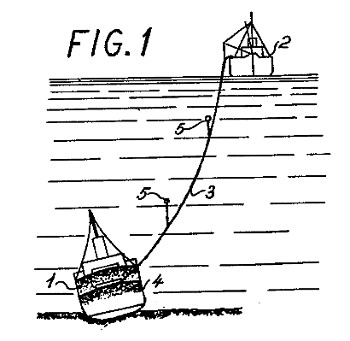
On Sept. 14, 1964, a Kuwaiti freighter capsized, drowning its cargo of sheep and threatening to contaminate the drinking water of Kuwait City. To raise the ship quickly, Danish inventor Karl Krøyer proposed using a tube to fill it with buoyant bodies. Accordingly, 27 million plastic balls were airlifted from Berlin and pumped into the freighter’s hold, and on Dec. 31 the ship rose, saving the insurance company nearly $2 million.
Krøyer patented his technique in the United Kingdom and Germany, but (the story is told) the Dutch application was rejected because a Dutch examiner found the 1949 Donald Duck comic The Sunken Yacht, by Carl Barks, in which Donald and his nephews raise a yacht by filling it with ping-pong balls.
Ping-pong balls are buoyant, and the ducks used a tube to feed them into the yacht, so the Dutch office ruled that this destroys the novelty of Krøyer’s invention — it may be just a comic book, but it had made the essential idea public 15 years before Krøyer tried to claim it.
No one quite seems to know whether this story is true — Krøyer, his patent attorney, and the examiner have now passed away; the documentation was destroyed years ago; and the grounds for the Dutch rejection aren’t clear. But it still makes a vivid example for intellectual property lawyers.


In Dante’s Inferno, a sign above the gate to hell reads LASCIATE OGNI SPERANZA, VOI CH’ENTRATE.
There are many ways to translate this (Robert Ripley claimed to find 100), but a common one is ABANDON YE ALL HOPE WHO ENTER HERE.
By an unlikely coincidence, this yields ABANDON Y.A.H.W.E.H.
(Discovered by Dave Morice.)

“There is nothing so wretched or foolish as to anticipate misfortunes. What madness it is in your expecting evil before it arrives!” — Seneca
“How much pain have cost us the evils which have never happened!” — Thomas Jefferson
“Our worst misfortunes never happen, and most miseries lie in anticipation.” — Balzac
“Ills that never happened, have chiefly made thee wretched.” — Martin Farquhar Tupper
“I remember the old man who said he had had a great many troubles in his life, but the worst of them never happened.” — James Garfield
“Let us be of good cheer, however, remembering that the misfortunes hardest to bear are those which never come.” — James Russell Lowell
“Real difficulties can be overcome; it is only the imaginary ones that are unconquerable.” — Theodore N. Vail