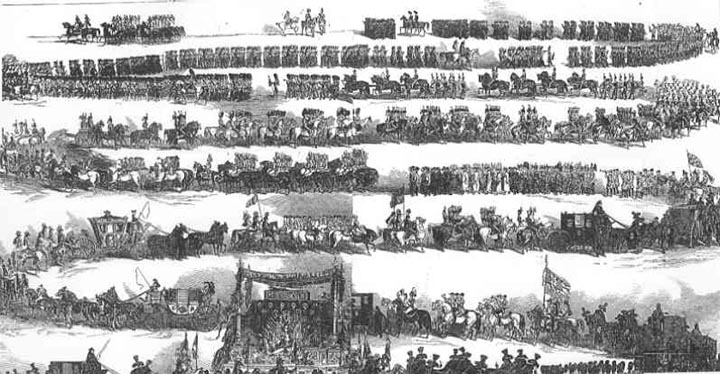
When the Duke of Wellington died in 1852, his funeral procession was watched by a crowd of 1.5 million people. To commemorate it, Henry Alken and George Augustus Sala painted a panorama fully 20 meters long, which was released the following year:
Wellington had certainly earned some distinction — his style was proclaimed in the London Gazette:
Duke and Marquess of Wellington,
Marquess Douro, Earl of Wellington,
Viscount Wellington and Baron Douro,
Knight of the Most Noble Order of the Garter,
Knight Grand Cross of The Most Honourable Order of the Bath,
One of Her Majesty’s Most Honourable Privy Council, and
Field Marshal and Commander-in-Chief of Her Majesty’s Forces.
Field Marshal of the Austrian Army,
Field Marshal of the Hanoverian Army,
Field Marshal of the Army of the Netherlands,
Marshal-General of the Portuguese Army,
Field Marshal of the Prussian Army,
Field Marshal of the Russian Army,
and
Captain-General of the Spanish Army.
Prince of Waterloo, of the Kingdom of the Netherlands, Duke of Ciudad Rodrigo
and Grandee of Spain of the First Class.
Duke of Victoria, Marquess of Torres Vedras, and Count of Vimiera in Portugal.
Knight of the Most Illustrious Order of the Golden Fleece, and of the Military Orders
of St. Ferdinand and of St. Hermenigilde of Spain.
Knight Grand Cross of the Orders of the Black Eagle and of the Red Eagle of Prussia.
Knight Grand Cross of the Imperial Military Order of Maria Teresa of Austria.
Knight of the Imperial Orders of St. Andrew, St. Alexander Newski, and St. George of Russia.
Knight Grand Cross of the Royal Portuguese Military Order of the Tower and Sword.
Knight Grand Cross of the Royal and Military Order of the Sword of Sweden.
Knight of the Order of St. Esprit of France.
Knight of the Order of the Elephant of Denmark.
Knight Grand Cross of the Royal Hanoverian Guelphic Order.
Knight of the Order of St. Januarius and of the Military Order of St. Ferdinand and
of Merit of the Two Sicilies.
Knight or Collar of the Supreme Order of the Annunciation of Savoy.
Knight Grand Cross of the Royal Military Order of Maximilian Joseph of Bavaria.
Knight of the Royal Order of the Rue Crown of Saxony,
Knight Grand Cross of the Order of Military Merit of Wurtemberg.
Knight Grand Cross of the Military Order of William of the Netherlands.
Knight of the Order of the Golden Lion of Hesse Cassel,
and
Knight Grand Cross of the Orders of Fidelity and of the Lion of Baden.
(Thanks, James.)