“Personalize your sympathies; depersonalize your antipathies.” — W.R. Inge
Author: Greg Ross
Ruly English
In 1957, the U.S. Patent Office wanted to design a computer that could track down earlier references to an idea submitted by an inventor. This is difficult, because patents are described in ordinary English, which uses many ambiguous and imprecise terms. The word glass, for instance, refers to a material, but also to any number of things made of that material, and even to objects that have nothing to do with glass, such as plastic eyeglasses and drinking glasses.
To solve this problem, engineer-lawyer Simon M. Newman planned a synthetic language called Ruly English that gave one and only one meaning to each word. In ordinary English the preposition through has at least 13 meanings; Newman proposed replacing each of them with a new Ruly term with a single meaning. The Ruly word howby, for example, means “mode of proximate cause.” It might replace the unruly terms by(take by force) or with (to kill with kindness) or through (to cure through surgery), but it always has the same basic sense.
Newman had to coin other terms to take account of differing points of view. A watch spring and a bridge girder are both flexible to some degree, but using the word flexible to describe both would leave a computer at a loss as to how they compare. Newman coined the Ruly word resilrig to cover the whole scale, from extreme flexibility to extreme rigidity, adding prefixes such as sli (slightly) and sub (substantially). So in Ruly English a bridge girder would be sliresilrig and a watch spring subresilrig. A computer that knew these terms would not be confused into thinking that a thin bridge girder was more flexible than a rigid watch spring.
“Humans are not expected to read or speak Ruly English,” noted Time in 1958. “To them, unruly English will always be more ruly.”
(Newman describes his plan briefly here. I don’t know how far he got.)
“The Kiss”
“I saw you take his kiss!” “‘Tis true.”
“O modesty!” “‘Twas strictly kept:
He thought me asleep — at least, I knew
He thought I thought he thought I slept.”
— Coventry Patmore
Podcast Episode 102: The Bunion Derby

In 1928, 199 runners set out on a perilous 3,400-mile footrace across America, from Los Angeles to Chicago and on to New York. The winner would receive $25,000 — if anyone finished at all. In this week’s episode of the Futility Closet podcast we’ll follow the Trans-American Footrace, better known as the Bunion Derby, billed as the greatest footrace the world had ever known.
We’ll also learn some creepy things about spiders and puzzle over why one man needs three cars.
Loss

From C.S. Lewis’ A Grief Observed, a collection of reflections on the loss of his wife, Joy, in 1960:
It is hard to have patience with people who say ‘There is no death’ or ‘Death doesn’t matter.’ There is death. And whatever is matters. And whatever happens has consequences, and it and they are irrevocable and irreversible. You might as well say that birth doesn’t matter. I look up at the night sky. Is anything more certain that in all those vast times and spaces, if I were allowed to search them, I should nowhere find her face, her voice, her touch? She died. She is dead. Is the word so difficult to learn? …
Talk to me about the truth of religion and I’ll listen gladly. Talk to me about the duty of religion and I’ll listen submissively. But don’t come talking to me about the consolations of religion or I shall suspect that you don’t understand.
He published it originally under the pseudonym N.W. Clerk, a pun on the Old English for “I know not what scholar.”
Fair Enough

Joseph Grinnell’s Game Birds of California (1918) notes that the avocet is known as the lawyer bird because of “its long bill and its oft-repeated vociferations.”
“The Pythagorean Curiosity”
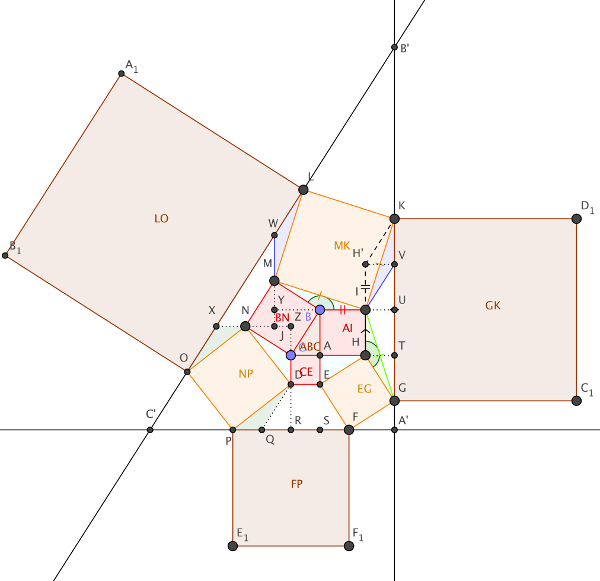
Here’s the item I mentioned in Episode 99 of the podcast — New York City engineer John Waterhouse published it in July 1899. It’s not a proof of the Pythagorean theorem, as I’d thought, but rather a related curiosity. It made a splash at the time — the Proceedings of the American Society of Civil Engineers said it “interested instructors of geometry all over the country, bringing many letters of commendation to him from prominent teachers.” Listener Colin Beveridge has been immensely helpful in devising the diagram above and making sense of Waterhouse’s proof as it appears on page 252 of Elisha Scott Loomis’ 1940 book The Pythagorean Proposition. Click the diagram to enlarge it a bit further.
- Red squares BN = AI + CE — Pythagoras’s theorem
- Blue triangles AEH, CDN, BMI are all equal in area to ABC, reasoning via X and Y and base sides.
- Green angles GHI and IBM are equal and green triangle GHI is congruent to IBM (side angle side), so IG = IK = IM. IH′K is congruent to IHK as angle HIK = angle HIG and the adjacent sides correspond. This means G and K are the same distance from the line HH′, so GK is parallel to HI. Similarly, DE is parallel to PF and MN is parallel to LO.
- GK = 4HI, because TU=HI, TG = AH (HTG congruent to EAH) and UK = UG (symmetry). Similarly, PF = 4DE. Dark blue triangles IVK and LWM are equal, so WM = VK. Similarly, OX = QD (dark green triangles PQD and NXO are congruent). Also, WX=MJ and XN=NJ, so M and N are the midpoints of WJ and XJ. That makes WX=2MN, so LO = 4MN.
- Each of the trapezia we just looked at (HIKG, OLMN and PFED) have five times the area of ABC.
- The areas of orange squares MK and NP are together five times EG. This is because:
- the square on MI is (the square on MY) + (the square on IY) = (AC2) + (2AB)2 = 4AB2 + AC2.
- the square on ND is (the square on NZ) + (the square on DZ) = (AB2) + (2AC)2 = 4AC2 + AB2
- the sum of these is 5(AB2 + AC2) = 5BC2, and BC = HE.
- A′S = A′T, so A′SAT is a square and the bisector of angle B′A′C′ passes through A. However, the bisectors of angle A′B′C′ and A′C′B′ do not pass through B and C (resp.) [Colin says Waterhouse’s reasoning for this is not immediately clear.]
- Square LO = square GK + square FP, as LO = 4AC, GK = 4AB and FP = 4BC.
- [We’re not quite sure what Waterhouse means by “etc. etc.” — perhaps that one could continue to build squares and triangles outward forever.]
Boontling
Boonville, Calif., has a dwindling language all its own. “Boontling” grew up as a jargon among residents of Anderson Valley around the turn of the 20th century. It includes more than a thousand words and phrases but is dying out among the small population. A brief glossary:
applehead – a young girl
belhoon – a dollar
Bill Nunn – syrup
boshin’ – deer hunting
bucky walter – a pay telephone
can-kicky – angry
dicking – cheating at cards
forbes – a half dollar
glow worm – a lantern
greeley – a newspaper or reporter
harpin’ tidrick – a lengthy discussion
high pockets – a wealthy person
killing snake – working very hard
pearlin – light rain
skee – whiskey
tobe – tobacco
walter – a telephone
zeese – coffee
“A few of us try to keep our skills sharp on the teleef [telephone],” resident Bobby Glover told the San Francisco Chronicle in 2001. “We’re adding new words now that the old-timers are gone.”
Thanks to the efforts of a number of researchers, the jargon has been pretty well documented now — the Chronicle even managed to translate “Old Mother Hubbard”:
The old dame piked for the chigrel nook for gorms for her bahl belljeemer
The gorms had shied, the nook was strung, and the bahl belljeemer had neemer.
Twos and Tens
The Wikipedia page for 1024 gives a handy technique for estimating large powers of 2 in decimal notation. For exponents up to about 100,
210a+b ≈ 2b103a.
For example, 235 = 34359738368 ≈ 32 × 109 = 32000000000.
This works because 210 ≈ 103. 3a gives a good estimate of the number of digits for exponents up to 300.
(Thanks, Stephen.)
Bedtime Stories

In his 1948 book The Lost Art of Profanity, Burges Johnson quotes from an opinion by a Judge Hammond of the Supreme Judicial Court of Massachusetts:
The Watch and Ward Society of Boston years ago brought charges against a certain magazine for printing obscene matter, and my old friend the late Kendall Banning was forced to defend the publication. He felt sure that he could make a case, and during the train ride to Boston he had a sudden idea, and began jotting down such nursery rhymes as he could recall. Then he crossed out significant words and substituted asterisks. In court he asked permission to read these rhymes. They later appeared in a privately printed brochure which aroused delight or horror, according to the state of the reader’s mind. A mere sampling will serve here:
A dillar, a dollar
A ten-o’clock scholar,
What makes you *** so soon?
You used to *** at ten o’clock,
But now you *** at noon.Jack and Jill went up the hill
To *******
Jack fell down and broke his ***
And Jill came tumbling after.
Johnson says that the courtroom broke out in laughter and that Banning had made his point.
04/15/2016 UPDATE: Related (thanks, Mate):