Author: Greg Ross
None for All
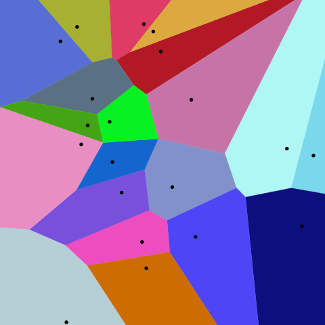
If a lion hunts a herd of antelope, what rules govern the herd’s behavior? One intriguing possibility is known as selfish herd theory: Rather than acting to benefit the group as a whole, each member positions itself so that there’s at least one other animal between it and the predator. This produces a pattern known as a Voronoi tesselation — if each dot in the diagram above is an antelope, then the surrounding colored region is the area that’s closer to that antelope than to any of its neighbors. If a lion enters your cell, then you’re the antelope that’s going to get eaten.
This understanding helps to explain some herd behavior. Each animal wants to make its “domain of danger” as small as possible and to be as far as possible from the predator. Dominant animals tend to get prime positions near the center, subordinate animals get pushed to the fringes, and the whole formation evolves continuously as predator and prey move about.
Studies have shown that groups of fiddler crabs tend to take up Voronoi patterns fairly quickly when a predator first appears, and to huddle together when the danger increases as each tries to reduce its surrounding polygon. This actually leads some to move toward the predator as they try to reach the center and put others between the hunter and themselves. Those that violate the movement rules tend to get picked off, which reinforces the evolutionary strength of the strategy.
Unquote
“An ignorant person is one who doesn’t know what you have just found out.” — Will Rogers
Skyward

An aviators’ drinking song from World War I, from James Gilbert’s 1978 anthology Skywriting:
A young aviator lay dying
At the end of a bright summer’s day.
His comrades had gathered around him
To carry his fragments away.
The aeroplane was piled on his wishbone,
His Lewis was wrapped round his head,
He wore a spark plug in each elbow,
‘Twas plain he would shortly be dead.
He spat out a valve and a gasket
As he stirred in the sump where he lay,
And then to his wondering comrades
These brave parting words did he say:
“Take the manifold out of my larynx
And the butterfly valve off my neck.
Remove from my kidneys the camrods;
There’s a lot of good parts in this wreck.
“Take the piston rings out of my stomach,
And the cylinders out of my brain.
Extract from my liver the crankshaft,
And assemble the engine again.
“Pull the longeron out of my backbone,
The turnbuckle out of my ear,
From the small of my back take the rudder —
There’s all of your aeroplane here.”
The Union-Closed Sets Conjecture
A family of sets is said to be union-closed if, given any two sets in the family, their union is as well. Here’s an example:
{}, {1,3}, {2}, {1,2,3}, {1,2,3,4}
Combine any two of those sets and you’ll get a member of the same family.
Now, provided our family is finite and consists of more than just the empty set, is there always an element that’s present in at least half of the sets? In the example above, the answer is yes: The element 2 appears in three of the five sets.
Will this always be the case? Strange to say, no one knows. Though the problem is almost absurdly simple to state, it has remained unsolved since Péter Frankl first posed it in 1979.
Henning Bruhn and Oliver Schaudt survey the state of the inquiry here. “The union-closed sets conjecture still has a bit of a journey ahead of it,” they conclude. “We hope it will be an exciting trip.”
(Thanks, Drake.)
In a Word

ocivity
n. laziness
Podcast Episode 93: The Old Flying Days
In the early days of English aviation, journalist C.C. Turner seemed to be everywhere, witnessing bold new feats and going on some harrowing adventures of his own. In this week’s episode of the Futility Closet podcast we’ll sample Turner’s record of Edwardian aviation, including his own clumsy first attempt to fly an airplane and a record-setting balloon voyage to Sweden.
We’ll also ponder the nuances of attempted murder and puzzle over a motel guest’s noisemaking.
As Advertised

The Museum of English Rural Life got a surprise on Wednesday — a 155-year-old mousetrap there managed to catch a mouse:
So, this retired rodent had managed to sneak past University of Reading security, exterior doors and Museum staff, and clambered its way up into our Store. Upon finding itself there it would have found the promised land; a mouse paradise laid before it full of straw, wood and textiles. Then, out of thousands of objects, it chose for its home the very thing designed to kill it some 150 years ago: a mouse trap.
The trap was patented in 1861; it bills itself as a “perpetual mouse trap” that “will last a lifetime.” More at the museum’s blog.
(Thanks, Djerrid.)
Foregone Conclusions

Here’s the opening of Alice’s Adventures in Wonderland:
Alice was beginning to get very tired of sitting by her sister on the bank, and of having nothing to do; once or twice she had peeped into the book her sister was reading.
Choose any of the first 12 words and tap your way forward in the sentence from that point, tapping one word for each letter. So, for example, if you choose the word Alice, which has five letters, you’d tap was, beginning, to, get, and land on very. Then do the same thing with that word, advancing four letters to land on by. If you keep this up you’ll always arrive at the word sister.
That’s from Martin Gardner; the same trick works with “Twinkle, Twinkle, Little Star,” the opening of the Bible, and countless other texts.
It’s less surprising than it seems — it’s based on a principle called the Kruskal count, proffered originally by Rutgers physicist Martin Kruskal as a card trick. In each case various tributaries merge into a common stream that arrives at a predictable destination. Here’s an analysis (PDF).
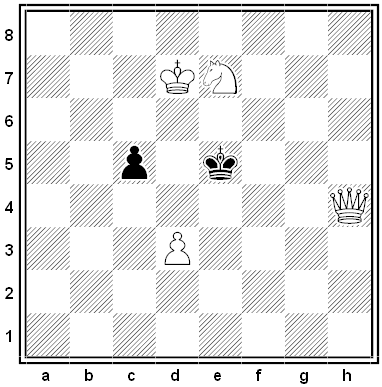
The Dark Side

In a certain chess position, each row and each column contains an odd number of pieces. Prove that the total number of pieces on black squares is an even number.