A problem from the 1973 American High School Mathematics Examination:
In this equation, each of the letters represents uniquely a different digit in base 10:
YE × ME = TTT.
What is E + M + T + Y?
A problem from the 1973 American High School Mathematics Examination:
In this equation, each of the letters represents uniquely a different digit in base 10:
YE × ME = TTT.
What is E + M + T + Y?

Being an angel is hard work. In his 1926 essay “On Being the Right Size,” J.B.S. Haldane writes, “An angel whose muscles developed no more power weight for weight than those of an eagle or a pigeon would require a breast projecting for about four feet to house the muscles engaged in working its wings, while to economize in weight, its legs would have to be reduced to mere stilts.”
And this takes no account of the weight of the harp. In The Book of the Harp, John Marson notes that gold is about 10 times heavier than willow, once the favorite wood of Celtic harp makers. He calculates that a harp of gold would weigh 120 pounds, far more than the 70-80 pounds of the largest pedal harp.
Should we worry about this? Let us not forget that it was angels who destroyed Babylon for its people’s wrongdoings. In the Book of Revelation, chapter 18, verse 21 tells us: “And a mighty angel took up a stone like a great millstone, and cast it into the sea, saying, ‘Thus with violence shall that great city of Babylon be thrown down.'”
This becomes a public health matter. Even if harps aren’t thrown at us deliberately by vengeful angels, Marson writes, “there is always the danger of one being dropped accidentally from a great height, resulting in the kind of damage caused on occasion by meteorites — unless, of course, the Bible is indeed correct after all, and angels do not play harps.”
See Hesiod’s Anvil.
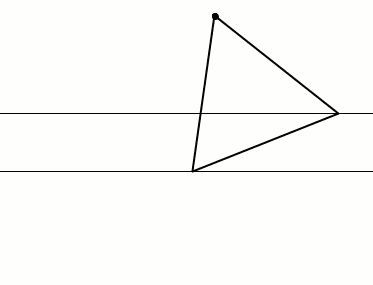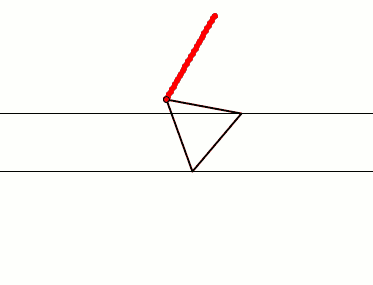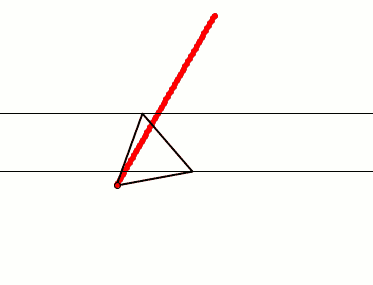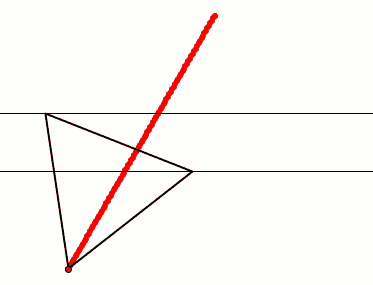
A pleasing fact from David Wells’ Archimedes Mathematics Education Newsletter:
Draw two parallel lines. Fix a point on one line and move a second point along the other line. If an equilateral triangle is constructed with these two points as two of its vertices, then as the second point moves, the third vertex of the triangle will trace out a straight line.
Thanks to reader Matthew Scroggs for the tip and the GIF.

In the 1920s Bata Kindai Amgoza ibn LoBagola toured the United States and Europe to share the culture of his African homeland with fascinated audiences. The reality was actually much more mundane: His name was Joseph Lee and he was from Baltimore. In this week’s episode of the Futility Closet podcast we’ll tell the curious story of this self-described “savage” and trace the unraveling of his imaginative career.
We’ll also dump a bucket of sarcasm on Duluth, Minnesota, and puzzle over why an acclaimed actor loses a role.
In March 1999, fisherman Steve Gowan was fishing for cod off the coast of Essex when he dredged up a green ginger beer bottle with a screw-on rubber stopper. Inside he found a note:
Sir or madam, youth or maid,
Would you kindly forward the enclosed letter and earn the blessing of a poor British soldier on his way to the front this ninth day of September, 1914.
Signed
Private T. Hughes
Second Durham Light Infantry.
Third Army Corp Expeditionary Force.
The enclosed letter read:
Dear Wife,
I am writing this note on this boat and dropping it into the sea just to see if it will reach you. If it does, sign this envelope on the right hand bottom corner where it says receipt. Put the date and hour of receipt and your name where it says signature and look after it well. Ta ta sweet, for the present.
Your Hubby.
Private Thomas Hughes, 26, of Stockton-on-Tees, had dropped the bottle into the English Channel in 1914 as he left to fight in France. He was killed two days afterward. His wife Elizabeth and daughter moved to New Zealand, where Elizabeth died in 1979. Gowan delivered the letter to the daughter, Emily Crowhurst, in Auckland that May. Two years old when her father had left for the war, she was now 86. She said, “It touches me very deeply to know … that his passage reached a goal. I think he would be very proud it had been delivered. He was a very caring man.”
Theoretical physicist Paul Dirac offered this example to show that some objects return to their original state after two full rotations, but not after one.
Hold a cup of water in one hand and rotate it through 360 degrees (in either direction). You’ll have to contort yourself to accomplish this without spilling any water, but if you continue rotating the cup another 360 degrees in the same direction, you’ll find that you return to your original state.
The same principle can be demonstrated using belts. In the video below, the square goes through two full rotations and we find that the belts have returned to their original state. This would not be the case after a single rotation. (Here two belts are attached to the square, but the trick works with any number of belts.)
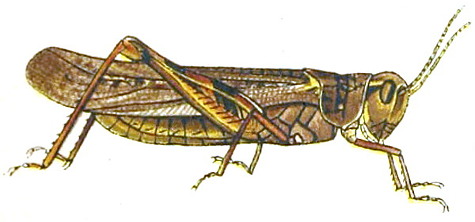
On June 15, 1875, physician Albert Childs was standing outside his office in Cedar Creek, Nebraska, when he saw the horizon darken. At first he was hopeful for some needed rain, but then he realized that the cloud was moving under its own power.
“And then suddenly it was on him, a trillion beating wings and biting jaws,” writes entomologist Steve Nicholls in Paradise Found (2009). It was an unusually huge swarm of Rocky Mountain locusts descended from the mountains. Stunned, Childs set about estimating its size:
Using the telegraph, he sent messages up and down the line and found the swarm front to be unbroken for 110 miles. With his telescope he estimated the swarm to be over half a mile deep, and he watched it pass for ‘five full days.’ He worked out that the locusts were traveling at around fifteen miles an hour and came up with the astonishing fact that the swarm was 1,800 miles long. This swarm covered 198,000 square miles, or, if it was transposed on to the east coast, it would have covered all the states of Connecticut, Delaware, Pennsylvania, Maryland, Maine, Massachusetts, New Jersey, New York, New Hampshire, Rhode Island, and Vermont.
“Albert Childs had recorded the largest ever swarm — the biggest aggregation of animals ever seen on planet Earth,” Nicholls writes. University of Wyoming entomologist Jeffrey Lockwood calls it the “Perfect Swarm.”
Another interesting item from James Tanton’s Mathematics Galore! (2012):
Write down a sequence of positive integers that never decreases. The list can include duplicates. As an example, here’s a list of primes:
2, 3, 5, 7, 11, 13
Call the sequence pn. Now, a “frequency sequence” records the number of members less than 1, less than 2, and so on. For the list of primes above, the frequency sequence is:
0, 0, 1, 2, 2, 3, 3, 4, 4, 4, 4, 5, 5, 6
Pleasingly, the frequency sequence of the frequency sequence of pn is pn. That is, if we take the frequency sequence of the list 0, 0, 1, 2, 2, 3, 3, 4, 4, 4, 4, 5, 5, 6 above, we get 2, 3, 5, 7, 11, 13 again.
Now add position numbers to each of the two lists, pn and its frequency sequence — that is, add 1 to the first element of each, 2 to the second, and so on. With the primes that gives us:
Pn: 3, 5, 8, 11, 16, 19 …
Qn: 1, 2, 4, 6, 7, 9, 10, 12, 13, 14, 15, 17, 18, 20 …
These two sequences will always be complementary — all the counting numbers appear, but they’re split between the two sequences, with no duplicates.
For what it’s worth, here’s a dance from the 1780s:
It’s “A Sailor Hornpipe — Old Style,” by John Durang, George Washington’s favorite dancer. Durang taught it to his son Charles, who reproduced it in a study of theatrical dancing published in 1855, which is how it comes down to us.
The terminology is influenced by French ballet, but already it incorporates innovations such as “shuffles”; in time the hornpipe would evolve into modern tap dancing. In Tap Roots, Mark Knowles writes, “It is believed that the ‘whirligig, with beats down’ is similar to a renversé turn such as the kind later done by the tap dancing film star Eleanor Powell.”
(From Julian Mates, The American Musical Stage Before 1800, 1962.)
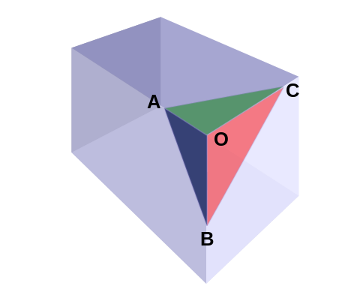
French mathematician Jean Paul de Gua de Malves discovered this three-dimensional analogue of the Pythagorean theorem in the 18th century.
If a tetrahedron has a right-angled corner (such as the corner of a cube), then the square of the area of the face opposite that corner is the sum of the squares of the areas of the other three faces.
Above,