
“Happiness is lost by criticizing it; sorrow by accepting it.” — Ambrose Bierce

“Happiness is lost by criticizing it; sorrow by accepting it.” — Ambrose Bierce
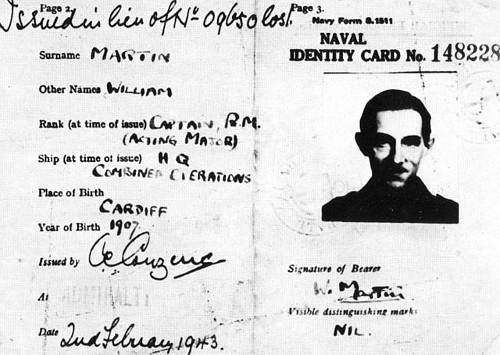
In 1942, Germany discovered a dead British officer floating off the coast of Spain, carrying important secret documents about the upcoming invasion of Europe. In this week’s episode of the Futility Closet podcast we’ll describe Operation Mincemeat, which has been called “the most imaginative and successful ruse” of World War II.
We’ll also hear from our listeners about Scottish titles and mountain-climbing pussycats and puzzle over one worker’s seeming unwillingness to help another.

For his James Bond Dossier (1965), Kingsley Amis went through the 12 Ian Fleming books in which the character M appears and found that they give “a depressingly unvaried picture of what he’s like to be with, or anyway work for.” M’s demeanor or voice is described as:
Amis says this “divides out as an irascibility index of just under 4.6 per book.”
The character seems to be a composite of several people whom Fleming had known, but he appears to be modeled most closely on Rear Admiral John Godfrey (above), Fleming’s superior at the Naval Intelligence Division during World War II. After Fleming’s death, Godfrey complained, “He turned me into that unsavoury character, M.”

Suppose you hire two proofreaders to go through the same manuscript independently. The first reports A mistakes, the second reports B mistakes, and C mistakes are reported by both. How can you estimate how many errors remain undiscovered?
Let M be the total number of mistakes in the manuscript. Then the number undiscovered by the two proofreaders is M – (A + B – C). Let p and q be the probabilities that the first and second proofreaders, respectively, notice any given mistake. Then A ≈ pM and B ≈ qM. And because they work independently, the chance that they both find a given mistake is C ≈ pqM.
But now
and the number of misprints that remain unnoticed is just
This means that as long as the proofreaders work independently, you can estimate the number of errors they’ve overlooked without even knowing how skillful they are. If they find a large number of mistakes in common but relatively few independently, then the manuscript is probably relatively clean. But if they generate large independent lists of errors with few in common, there are probably many mistakes remaining to be found (which matches our intuition).
(George Pólya, “Probabilities in Proofreading,” American Mathematical Monthly 83:1 [January 1976], 42.)
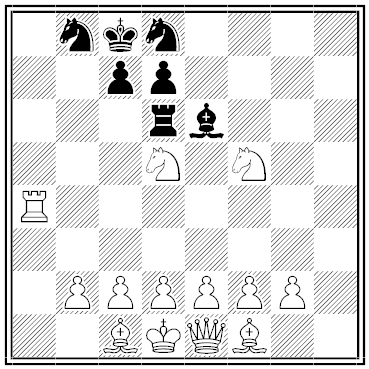
White to play and mate on his first move.
(Don’t spend too much time on this — it’s a bit of a trick.)

hallucar
adj. pertaining to the big toe
In 1856, 10-year-old Ellen Terry was just about to give Puck’s final speech in A Midsummer Night’s Dream when a stagehand closed a trapdoor on her foot, breaking her toe. She screamed, but manager Ellen Kean offered to double her salary if she finished the play. So, supported by Kean on one side and her sister Kate on the other, she delivered the following soliloquy:
If we shadows have offended (Oh, Katie, Katie!)
Think but this, and all is mended, (Oh, my toe!)
That you have but slumbered here,
While these visions did appear. (I can’t, I can’t!)
And this weak and idle theme,
No more yielding but a dream, (Oh, dear! oh, dear!)
Gentles, do not reprehend; (A big sob)
If you pardon, we will mend. (Oh, Mrs. Kean!)
“How I got through it, I don’t know!” she wrote in her 1908 memoir. “But my salary was doubled — it had been fifteen shillings, and it was raised to thirty — and Mr. Skey, President of Bartholomew’s Hospital, who chanced to be in a stall that very evening, came round behind the scenes and put my toe right. He remained my friend for life.”
In the December 2012 issue of 1 Across magazine, longtime crossword composer John Graham included a special instruction above one of his puzzles:
“I have 18dn of the 19; no 27, just 13 15; no 2 or 6 or 1dn 26 yet — plenty of 10, though I wouldn’t have chosen the timing.”
Solvers discovered that 18 down was CANCER and 19 across was OESOPHAGUS. The full message read:
“I have CANCER of the OESOPHAGUS; no CHEMOTHERAPY, just PALLIATIVE CARE; no NARCOTIC or STENT or MACMILLAN NURSE yet — plenty of MERRIMENT, though I wouldn’t have chosen the timing.”
The puzzle was reprinted as cryptic crossword No. 25,842 in the Guardian the following month.
“It seemed the natural thing to do somehow,” Graham said. “It just seemed right.” He died in November 2013, and the Guardian published a tribute crossword to remember him.
(Thanks, Anthony.)
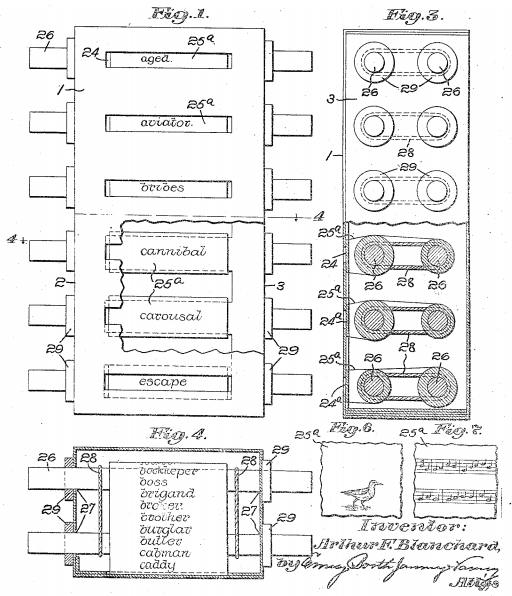
Here’s a curious invention from 1916, in the early days of motion pictures: It’s a machine designed to suggest plot ideas by randomly juxtaposing ideas. Words, pictures, and even bars of music are printed on paper rollers, and the writer turns these to present six elements that form the basis of a story.
In the example above, the machine presents the words aged, aviator, bribes, cannibal, carousal, and escape. “These particular words readily suggest, for instance, that an aged aviator after flying through the air on a long trip, lands finally on a desolate island where he is met by a cannibal, whom he is forced to bribe to secure his safety. After an interim which is full of possibilities as a basis of a story, a carousal ensues following which the aviator escapes.”
Inventor Arthur Blanchard says that this technique can be used to inspire any fictional work, from a cartoon to a song, but he patented it specifically as a “movie writer.” Whether it inspired any movies I don’t know.
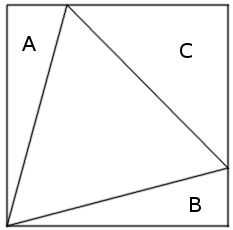
If an equilateral triangle is inscribed in, and has a common vertex with, a rectangle, as shown above, then areas A + B = C.
If a triangle with angles α, β, γ is inscribed in, and has a common vertex with, a rectangle, as shown below, and if the right triangles opposite α, β, γ have areas A, B, C, respectively, then A cot α + B cot β = C cot γ.
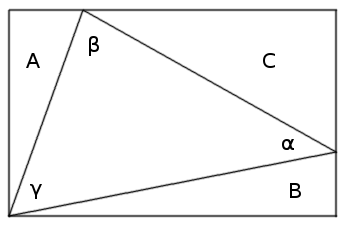
Somewhat related: A Curious Equality.
(Tom M. Apostol and Mamikon Mnatsakanian, “Triangles in Rectangles,” Math Horizons 5:3 [February 1998], 29-31.)

On Sept. 26, 1901, 13-year-old Fleetwood Lindley was attending school in Springfield, Ill., when his teacher handed him a note: His father wanted him urgently. He rode his bicycle to the Oak Ridge cemetery two miles out of town and found his father, Joseph, in the memorial hall of Abraham Lincoln’s tomb. The assassinated president, now 36 years dead, was being transferred to a new resting place, and a small group of caretakers had decided to open his coffin to confirm his identity.
The casket had been laid across a pair of sawhorses. A pair of workmen used a blowtorch to unseal the lead panel that covered Lincoln’s upper body, and the small group peered in.
Afterward the coffin was lowered into a hole 10 feet deep, encased in a cage of steel bars, and buried under tons of concrete. Over the years, as the other witnesses passed away, Lindley became the last living person to have looked on Lincoln’s body.
“His face was chalky white,” he remembered for a Life reporter in 1963, three days before his own death. “His clothes were mildewed. And I was allowed to hold one of the leather straps as we lowered the casket for the concrete to be poured.”
“I was not scared at the time, but I slept with Lincoln for the next six months.”