
decubation
n. the action of lying down
decubitus
n. the manner or posture of lying in bed

decubation
n. the action of lying down
decubitus
n. the manner or posture of lying in bed
Trap a circle inside a square and it can turn happily in its prison — a circle has the same breadth in any orientation.
Perhaps surprisingly, circles are not the only shapes with this property. The Reuleaux triangle has the same width in any orientation, so it can perform the same trick:
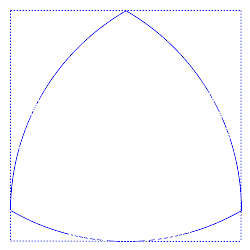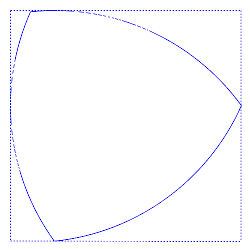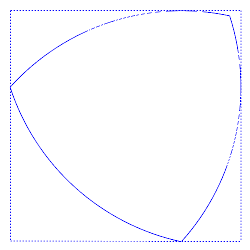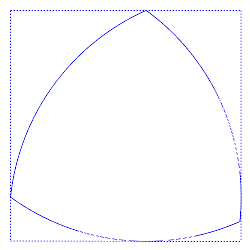
In fact any square can accommodate a whole range of “curves of constant width,” all of which have the same perimeter (πd, like the circle). Some of these are surprisingly familiar: The heptagonal British 20p and 50p coins and the 11-sided Canadian dollar coin have constant widths so that vending machines can recognize them. What other applications are possible? In the June 2014 issue of the Mathematical Intelligencer, Monash University mathematician Burkard Polster notes that a curve of constant width can produce a bit that drills square holes:
… and a unicycle with bewitching wheels:
The self-accommodating nature of such shapes permits them to take part in fascinating “dances,” such as this one among seven triangles:
This inspired Kenichi Miura to propose a water wheel whose buckets are Reuleaux triangles. As the wheel turns, each pair of adjacent buckets touch at a single point, so that no water is lost:
Here’s an immediately practical application: Retired Chinese military officer Guan Baihua has designed a bicycle with non-circular wheels of constant width — the rider’s weight rests on top of the wheels and the suspension accommodates the shifting axles:
(Burkard Polster, “Kenichi Miura’s Water Wheel, or the Dance of the Shapes of Constant Width,” Mathematical Intelligencer, June 2014.)
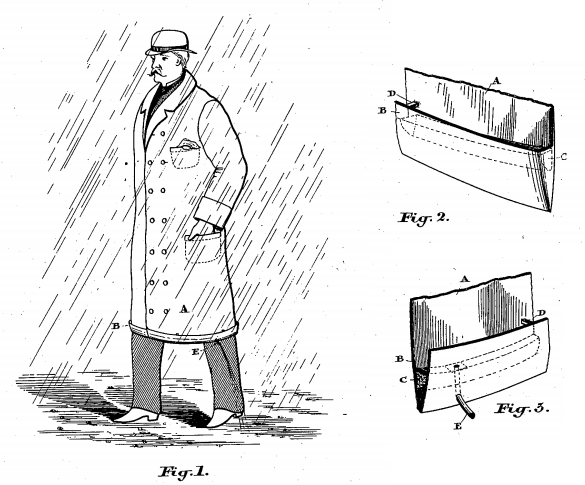
Torontonian John Maguire wasn’t satisfied with the standard raincoat in 1883, so he added a gutter:
The object of the invention is to provide a water-proof coat which can be worn in rainy weather without the wearer’s leg being made wet from water dripping off the skirt of the coat; and it consists of a water-proof coat having the bottom edge of its skirt turned up, forming a trough or channel to receive the water flowing on the surface of the coat, suitable provision being made to carry off the water away from the legs of the wearer of the garment.
“Although the coat is specially designed for gentlemen’s use, it will of course be understood that ladies’ coats may be similarly made.”

In May 1905 British MP Sir Gilbert Parker insisted that he had seen the astral body of Sir Crane Rasch in the House of Commons while Rasch was ill at home.
Sir Arthur Hayter supported him: “I beg to say that I not only saw Sir Carne Rasch myself sitting below the gangway but I called him to the attention of Sir Henry Campbell-Bannerman, with whom I was talking on the front opposition bench, saying I wondered why all the papers had inserted notices of Sir Carne’s illness while he was sitting opposite, apparently quite well. Sir Henry replied that he hoped his illness was not catching.”
Rasch declared later that he had never left his room.
“It seems that this is not the first instance of the sort that has occurred in the House,” noted the New York Sun. “In 1897 Mr. O’Connor, an Irish member, went to Ireland to be present at the deathbed of one of his parents. Swift McNeill saw his wraith in his usual seat on the third opposition bench. It was also seen from the press gallery.”
Albert Szent-Györgyi, who knew a lot about maps
according to which life is on its way somewhere or other,
told us this story from the war
due to which history is on its way somewhere or other:
The young lieutenant of a small Hungarian detachment in the Alps
sent a reconnaissance unit out into the icy wasteland.
It began to snow
immediately, snowed for two days and the unit
did not return. The lieutenant suffered: he had dispatched
his own people to death.
But the third day the unit came back.
Where had they been? How had they made their way?
Yes, they said, we considered ourselves
lost and waited for the end. And then one of us
found a map in his pocket. That calmed us down.
We pitched camp, lasted out the snowstorm and then with the map
we discovered our bearings.
And here we are.
The lieutenant borrowed this remarkable map
and had a good look at it. It was not a map of the Alps
but of the Pyrenees.
Goodbye now.
— From Miroslav Holub, Notes of a Clay Pigeon, reprinted in G.Y. Craig and E.J. Jones, A Geological Miscellany, 1982.
Football fans found an unlikely oracle during the 2008 European championship: an octopus named Paul. Before each match his keepers at the Sea Life Centre in Oberhausen, Germany, would lower two boxes of food into his tank, each bearing the flag of an upcoming competitor. Surprisingly, Paul correctly chose the winner in four of Germany’s six games.
When some observers expressed skepticism, Paul went on to pick the winners of all seven of Germany’s World Cup games in 2010, as well as the final between Spain and the Netherlands, giving him an overall success rate of 85 percent.
Competitors sprang up around the world, including a Singaporean parakeet, a German parrot, and a saltwater crocodile named Dirty Harry, who predicted the result of Australia’s general election by snatching a chicken carcass dangling beneath a caricature of Prime Minister Julia Gillard. Maybe we should quit while we’re ahead.
(Thanks, Lauren.)
A problem from the 2000 Moscow Mathematical Olympiad:
Some of the cards in a deck are face down and some are face up. From time to time Pete draws out a group of one or more contiguous cards in which the first and last are both face down. He turns over this group as a unit and returns it to the deck in the position from which he drew it. Prove that eventually all the cards in the deck will be face up, no matter how Pete proceeds.

In this week’s episode of the Futility Closet podcast we’ll follow the dramatic 14,000-mile clipper ship race of 1866, in which five ships competed fiercely to be the first to London with the season’s tea.
We’ll also track the importance of mulch to the readers of the comic book Groo the Wanderer and puzzle over the effects of Kool-Aid consumption on a woman’s relationships.

Only three countries have not officially adopted the metric system: Liberia, Myanmar, and the United States.
In October 2013 Myanmar announced that it plans to make the switch.

I may as well just tell you a few of the things I like, and then, whenever you want to give me a birthday present (my birthday comes once every seven years, on the fifth Tuesday in April) you will know what to give me. Well, I like, very much indeed, a little mustard with a bit of beef spread thinly under it; and I like brown sugar — only it should have some apple pudding mixed with it to keep it from being too sweet; but perhaps what I like best of all is salt, with some soup poured over it. The use of the soup is to hinder the salt from being too dry; and it helps to melt it. Then there are other things I like; for instance, pins — only they should always have a cushion put round them to keep them warm. And I like two or three handfuls of hair; only they should always have a little girl’s head beneath them to grow on, or else whenever you open the door they get blown all over the room, and then they get lost, you know.
— Lewis Carroll, letter to Jessie Sinclair, Jan. 22, 1878