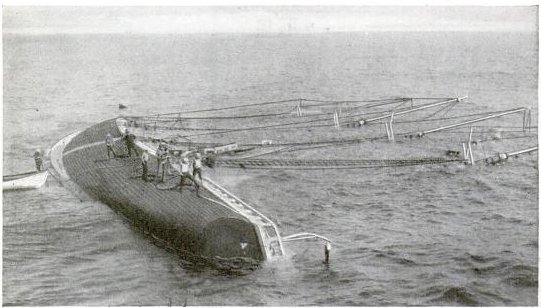
In 1929 the schooner A. Ernest Mills sank after a collision off the coast of North Carolina.
Four days later it bobbed to the surface again. Its cargo of salt had dissolved.

In 1929 the schooner A. Ernest Mills sank after a collision off the coast of North Carolina.
Four days later it bobbed to the surface again. Its cargo of salt had dissolved.

In July 1945, a former inhabitant of Poland’s Lódz ghetto, Avraham Benkel, returned to find his home in ruins. Lódz had been the last of 200 ghettos in German-occupied Poland, and as the war neared its conclusion tens of thousands of its inhabitants had been deported to death camps. When the Red Army reached it in January, only 877 survivors remained.
In the abandoned building next door to his own, Benkel found a book, François Coppée’s The Truly Rich, on whose endpages and margins an anonymous boy had kept a diary of his ordeal:
June 1, 1944: “I just finished my ‘loaf, which I had to have for eight days; today is the third. It may turn [out] to be lethal for me to behave in such a manner, but my will-power is so weakened and my ever increasing appetite so strong that I can’t help it. Is there no bread, dry unsavoury bread enough for me and my fellow-sufferers in this world?”
July 10, 1944: “I am exhausted, I have no more patience, my nerves are frayed. What I do have is an indescribable disgust toward the world and mankind, toward the masses and people, toward doctrines and dogmas. I do not believe, I do not believe in any change in the world, no! Anyone who can sink as low as the modern man has can be nothing more than an unsuccessful experiment of nature, which certainly regrets it!”
July 20, 1944: “I feel such a need to open my diary and to write in order to ease my bitter heart, about what hell we go through, how terribly we suffer. During the time when we had literally nothing to eat, we were willing to believe that the physical annihilation of men, women, and children of our nation had appeased the blond beast. But now it looks as if they have not had enough, and that they want to satisfy their thirst with the blood of the innocent.”
Almost nothing is known about the boy’s identity, age, background, schooling, or fate. Only the verb forms in his entries tell us that he was male. In all likelihood he was deported to Auschwitz as the Russians closed in.
“My God, why do you allow them to say that you are neutral?” he wrote in a final, undated entry. “Why will you not punish, with all your wrath, those who are destroying us? Are we the sinners and they the righteous? Is that the truth? Surely you are intelligent enough to understand that is it not so, that we are not the sinners and they are not the Messiah!”
(From Alexandra Zapruder, Salvaged Pages: Young Writers’ Diaries of the Holocaust, 2002.)

This puzzle was devised by W.T. Williams. The goal is to discover the age of Father Dunk’s mother-in-law (2 down), but the clues contain so much cross-reference and the grid so many interlocking solutions that practically the whole puzzle must be completed to find it.
The year is 1939. There are 20 shillings in a pound; 4840 square yards in an acre; a quarter of an acre in a rood; and 1760 yards in a mile.
ACROSS
1. Area in square yards of Dog’s Mead
5. Age of Martha, Father Dunk’s aunt
6. Difference in yards between length and breadth of Dog’s Mead
7. Number of roods in Dog’s Mead times 8 down
8. The year the Dunks acquired Dog’s Mead
10. Father Dunk’s age
11. Year of Mary’s birth
14. Perimeter in yards of Dog’s Mead
15. Cube of Father Dunk’s walking speed in miles per hour
16. 15 across minus 9 down
DOWN
1. Value in shillings per rood of Dog’s Mead
2. Square of the age of father Dunk’s mother-in-law
3. Age of Mary, father Dunk’s other daughter
4. Value in pounds of Dog’s Mead
6. Age of Ted, father Dunk’s son, who will be twice the age of his sister Mary in 1945
7. Square of the breadth of Dog’s Mead
8. Time in minutes it takes Father Dunk to walk 4/3 times round Dog’s Mead
9. The number which, when multiplied by 10 across, gives 10 down
10. See 9 down
12. Addition of the digits of 10 down plus 1
13. Number of years Dog’s Mead has been in the Dunk family
Hint: Start with 15 across, and keep your wits about you — most of the clues require some sort of insight or intelligent narrowing of the possible solutions; there’s very little mechanical plugging of numbers. With enough careful, dogged reasoning, it’s possible to complete the entire grid, but it’s stupendously hard.
Incontrovertibly the greatest nickname in history is The Snodgering Blee, Charles Dickens’ name for his eldest son, Charley. Some further inventive handles:
The Doubleday publishing company was founded in 1897 by Frank Nelson Doubleday, whose initials inevitably led Rudyard Kipling to dub him “effendi.” In Ogden Nash, Douglas M. Parker says this was “a nickname he would carry for his entire career.”

In All Quiet on the Western Front, Erich Maria Remarque describes a battlefield reflex that is “far quicker, much more sure, less fallible, than consciousness”:
A man is walking along without thought or heed;–suddenly he throws himself down on the ground and a storm of fragments flies harmlessly over him;–yet he cannot remember either to have heard the shell coming or to have thought of flinging himself down. But had he not abandoned himself to the impulse he would now be a heap of mangled flesh. It is this other, this second sight in us, that has thrown us to the ground and saved us, without our knowing how.
Apparently this was a real phenomenon, at least in World War I. Stephen Foot, a British officer who spent three years on the western front, writes in his 1934 memoir Three Lives:
It was about this time that I suddenly realised that I had become acclimatised to shell fire; not that I liked or had ceased to fear it, but that I was able to discriminate between a shell that was coming near and one that was far enough away not to worry about. Some officers recently arrived from England were responsible. They were in charge of one of the working parties, and while we were standing in a group, not far from Plug Street, we heard the whine of a German shell. I looked instinctively in the direction of the church to see the usual cloud of dust and stones, and at the same time I realised that the officers with me had ducked. I was certainly no braver than they were — quite the contrary; but my ear had somehow become attuned, so that as soon as the first sound was heard I knew approximately where the shell would burst.
Foot calls this sense “a great relief to one’s nervous system” and speculates that the worst cases of nervous breakdown occurred in men who didn’t have it. “One cannot explain it,” Remarque wrote. “If it were not so, there would not be one man alive from Flanders to the Vosges.”
In the summer of 1978, four men rowed a small boat into the deep water off Dassen Island, South Africa, to fish for barracuda. When mist overtook them, they weighed anchor and tried to return to shore, but visibility dropped so quickly that they were soon lost.
Mac Macgregor was in the bow, trying to peer through the mist, when he felt a bump on the right-hand side and discovered two dolphins there, repeatedly forcing the bow to the left, where two more dolphins were swimming.
“I realized that the dolphins’ odd behavior could be significant and shouted to Mr. [Kobus] Stander to steer to the left,” Macgregor said. “Mr. Stander pulled the tiller round wildly and we just managed to graze past the rocks.”
They continued some further distance through the mist, the dolphins continuing to force the prow to the left, and presently they just missed some further rocks — again on the right. “I was getting a strange feeling that we ought to leave our destiny to the dolphins,” Stander said, “since it was clear they had twice prevented us from running on to the rocks.”
The dolphins led the boat for half an hour until it entered calm water, then played around it briefly and disappeared. “When the mist cleared and the houses of Ysterfontein could be discerned, we were speechless,” Stander said. “We had intended going ashore at Dassen Island. We had never dreamed that the dolphins would guide us to Ysterfontein.”
In 1972, when her cabin cruiser sank in the Indian Ocean off Mozambique, a South African woman set out to swim the 25 miles to land. She was trailed by half a dozen sharks, attracted by a cut on her foot. But “as the sharks circled closer … two dolphins appeared at her side,” the New York Times reported. “The young woman, Yvonne Vladislavich, said that the dolphins guarded her against marauding sharks, escorted her as she swam and helped her stay afloat when her strength was failing.” They protected her until she was able to climb onto a buoy, from which she was later rescued.
(“Dolphins Rescue Fishermen,” South African Panorama, August 1978; “South African Reports a Rescue by Dolphins,” New York Times, Sept. 10, 1972.)
desticate
v. to squeak like a rat
latrate
v. to bark like a dog
curkle
v. to cry like a quail
barr
v. to utter an elephant’s cry
frantling
n. the noise made by peacocks
“When did the world begin and how?”
I asked a lamb, a goat, a cow:
“What’s it all about and why?”
I asked a hog as he went by:
“Where will the whole thing end and when?”
I asked a duck, a goose, a hen:
And I copied all the answers too,
A quack, a honk, an oink, a moo.
— Robert Clairmont
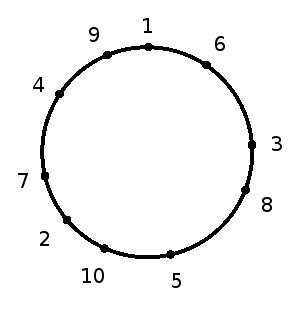
Draw a circle whose circumference is the golden mean. Choose a point and label it 1, then move clockwise around the circle in steps of arc length 1, labeling the points 2, 3, and so on. At each step, the difference between each pair of adjacent numbers on the circle is a Fibonacci number.