- Dorothy Parker named Alexander Woollcott’s apartment “Wit’s End.”
- Can you look at something and imagine it at the same time?
- 36850 = (36 + 8) × 50
- AGNOSTIC is an anagram of COASTING.
- “The errors of a man are what make him really lovable.” — Goethe
Author: Greg Ross
The Divestiture Puzzle

Suppose you own stock in a company that you believe has acted immorally. You want to sell the stock, but is this morally permissible? If owning the stock is wrong, then selling it to another person amounts to abetting an immoral act. The buyer might not feel the stock is tainted, but you do.
Even just renouncing ownership amounts to redistributing the stock’s value among the other stockholders, which increases their moral culpability. Is principled divestiture possible?
(Steven M. Cahn, “A Puzzle Concerning Divestiture,” Analysis 47:3 [1987], 175-176.)
Roll Call
Yet more unusual names of real people. Most of these are from the collection of Leland Hilligoss of the St. Louis Public Library, via Paul Dickson, A Collector’s Compendium of Rare and Unusual, Bold and Beautiful, Odd and Whimsical Names (1986). “As far as can be determined, all of the names are real and almost all were collected in North America and the British Isles”:
- Magdalena Babblejack
- Phoebe B. Peabody Beebe
- Sibyl Bibble
- Christian Bible
- Hiawatha Cathcart
- Tensil Cheesebrew
- Adeline Dingledine
- W. French Dingler
- Ed Ek
- JoAnn Floozbonger
- E. Vercel Fuglestad
- Cashmere Funkhouser
- L.E. Vontilzer Gleaves
- Felty Goosehead
- Icy Macy Hoober
- Zola G. Hooberry
- Square Horn Jr.
- Birdie T. Hospital
- Elizabeth Hogg Ironmonger
- Mingtoy Johnson
- Epluribus Kitchen
- Varnard P. Longhibler
- Channing Manning
- Duel Maroon
- Luch V. Moga
- Otis Muckenfuss
- Lester Ouchmoody
- Loveless Pelt
- Grace Pinkapank
- Evangelist Polite
- Curt Puke
- Burger Rocket
- Melon Roof
- Goolsby Scroggins
- Norval Sleed
- Craven Tart
- Eloise Tittlekitty
- Kong Vang
- Gwendolyne Winklepleck
- Clifteen Wooters
Boxing Day
Death row is overcrowded, so the warden proposes a radical solution. He places 100 boxes in a sealed room. Each contains a slip of paper bearing the name of one of the 100 prisoners on the row.
Each prisoner will enter the room by one door, open 50 boxes, and exit by another door. Unless every prisoner can discover his own name, all 100 will be executed.
The prisoners will be supervised by a guard. They cannot communicate with one another, and they must leave the room as they found it, but the group can prepare a plan in advance and post it on the wall of the room.
If they proceed at random, their chance of succeeding is 1/2100, or about 0.00000000000000000000000000000008. What should they do?
Skyline Trouble

In June 1978, a Princeton engineering student called structural engineer William LeMessurier with some worrying calculations. LeMessurier’s new Citicorp Tower, which had opened the previous year, was vulnerable to quartering winds — winds that blew from a 45-degree angle. On investigating, LeMessurier found also that the welded joints he had specified had been replaced with weaker bolted joints during construction. This meant that a strong wind could shear the bolts and topple a 59-story building into midtown Manhattan.
With hurricane season approaching, welders worked from 8 p.m. to 4 a.m. every night, reinforcing the building’s joints, and the Red Cross worked out an evacuation plan for the surrounding neighborhood. Because of a press strike at the time, many of these details came to light only 20 years later.
That year’s Hurricane Ella actually bore down on New York as the workers were finishing the job, but the storm veered out to sea before reaching the city. The welding was completed in October, and it’s now estimated that a storm strong enough to rock the tower will occur only once every 700 years.
Reversals
When Marshall Bean left the Army in 1965 after eight years’ service, he inverted his name to avoid his creditors. His new driver’s license and Social Security card read Naeb Llahsram.
Unfortunately, this fooled the Army, too, which drafted him back again in 1966. It took him more than a year to convince them he’d already served.
“All this is his own fault,” an Army spokesman told the Associated Press. “It would not have happened in the first place if he hadn’t spelled his name backwards.”
Accordion Commute
A puzzle by David Wells:
Every day I take the subway from Startville to Endville. Today I arrived at the Startville station to find that my train was just departing. I caught the next train to Endville, where I left the station at exactly the same time as if I had caught the first train. How did I manage this? The two trains traveled at the same speed, and I myself did not have to rush to make up the lost time.
Tock

How fast does time pass? We have no way to measure this. We can reply, helplessly, that it passes at one second per second, but this is not a rate of change — 1 second divided by 1 second is 1. Not 1 of anything, just 1.
“‘One’ can be an answer, right or wrong, to the questions ‘How many children had Lady Macbeth?’, ‘How many Gods are there?’, and ‘How many minutes do sixty seconds make?’,” writes Notre Dame philosopher Peter van Inwagen. “‘One’ can never be an answer, not even a wrong one, to any other sort of question — including those questions that ask ‘how fast?’ or ‘at what rate?’ Therefore, if time is moving, it is not moving at any rate or speed.”
(From his Metaphysics, 2002.)
Art Evergreen
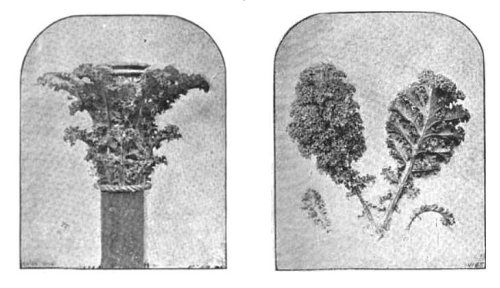
One of the most eminent architects in the kingdom once showed the accompanying photograph to a number of his colleagues. Had they ever seen such an exquisitely carved capital? They had not; and they said so. Then arose disputes as to the precise nature of the architecture. Finally sundry big wagers were made, and then the architect gravely proceeded to explain the structure of the column and its capital. This he did by producing his Malacca walking-stick and a few sprigs of succulent broccoli, such as are seen in Fig. 19. Naturally enough, however, after many abstruse disquisitions on mediæval architecture had been given on the subject of the mysterious pillar, this explanation of the photograph was received in silent disgust.
— William G. FitzGerald, “Some Curiosities of Modern Photography,” Strand, January 1895
“Sewing Machine Worked by a Dog”

From Gaston Tissandier’s Popular Scientific Recreations (1882). This is even worse than the dog treadmill, where at least the animal has the option to stand still — here he’s confined to a box on the side of a wheel, where, finding himself sliding downward, he’s perpetually forced to climb.
Tissandier says that the machine’s inventor, M. Richard of Paris, employed a large number of women working on sewing machines and conceived the idea of “quadrupedal motors” when he noticed the work was injuring their health. That was generous. “There is very little trouble or expense connected with the working, so a great saving is effected, as the dogs cost little, and are cheaply fed.” Perhaps he found a suitably ironic fate in the afterlife.
(Thanks, Richard.)
