
Australian philosopher J.J.C. Smart asks, “In what units is the rate of time flow to be measured? Seconds per — what?”

Australian philosopher J.J.C. Smart asks, “In what units is the rate of time flow to be measured? Seconds per — what?”
Visiting France in 1777, Benjamin Franklin received hundreds of inquiries from ardent Frenchmen seeking to join the American army. Finally he penned a “model of a letter of recommendation of a person you are unacquainted with”:
Sir.–The bearer of this, who is going to America, presses me to give him a letter of recommendation, though I know nothing of him, not even his name. This may seem extraordinary, but I assure you it is not uncommon here. Sometimes, indeed, one unknown person brings another equally unknown to recommend him; and sometimes they recommend one another! As to this gentleman, I must refer you to himself for his character and merits, with which he is certainly better acquainted than I can possibly be; I recommend him however to those civilities which every stranger, of whom one knows no harm, has a right to, and I request you will do him all the good offices and show him all the favour that, on acquaintance, you shall find him to deserve. I have the honour to be, &c.
It’s said that when Gustave Doré bought a villa on the outskirts of Paris, he had this notation inscribed over the entrance:
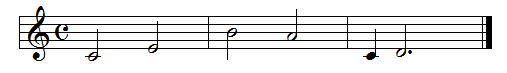
Do mi si la do re = “Domicile à Doré.” Get it?
Longfellow wrote, “Music is the universal language of mankind.”
We may safely suppose that the ocean ships of a hundred years hence will be driven by energy of some kind transmitted from the shores on either side. It is absolutely unquestionable that no marine engine in the least resembling what we know to-day can meet the requirements of the new age. The expense of driving a steamship increases in such a ratio to its size and speed that the economic limits of steam propulsion are foreseen. Probably the ships of A.D. 2000 will differ entirely in appearance from those we know. Just as road friction is the bugbear of the railway engineer, so water-resistance is the bugbear of the marine engineer. The ships of a hundred years hence will not lie in the water. They will tower above the surface, merely skimming it with their keels, and the only engines they will carry will be those which receive and utilise the energy transmitted to them from the power-houses ashore — perhaps worked by the force of the very tides of the conquered ocean itself.
— T. Baron Russell, A Hundred Years Hence, 1906
The cable guy is coming tomorrow between 8 a.m. and 4 p.m. Let’s bet on whether he turns up in the morning or the afternoon.
Both windows are four hours long, so as we sit here today, it seems rational to treat them as equally likely. But suppose you choose the morning. As the clock begins to tick, the morning window will gradually close, making the afternoon seem increasingly preferable. Though your present self regards the two eventualities as equally likely, it seems that your future self won’t. Should that affect your decision today?
(In my experience the guy never turns up at all, so perhaps that solves it.)
Hajek, Alan (2005), “The Cable Guy Paradox,” Analysis 65: 112-19.
This card curiosity is attributed to Lewis Carroll.
Lay down eight cards with these values:
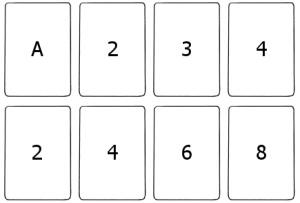
Now add the values in each column, find a card of that value in the deck, and place it on top of the lower card. Aces count as 1, jacks as 11, queens as 12, and kings as 13. Thus in the first column 1 + 2 = 3, so you’d place a 3 on top of the 2.
When you’ve done all four columns, repeat the process, placing a 4 on the 3, etc. If a sum is more than 13, subtract 13 from it (for example, Q + 7 = 19 – 13 = 6). When you’ve exhausted the deck you’ll have four kings in the bottom row. Place each of these piles on the card above it, then take up the packs from right to left.
Turn the deck face down again and deal 13 cards in a circle, making note of which was dealt first. Counting from that card, deal 13 more cards, placing them on every second pile (that is, piles 2, 4, 6, etc.) and continuing around the circle until 13 are dealt. Then deal 13 more cards, one onto each third pile (piles 3, 6, 9, etc.), and finish by dealing the last 13 cards, one onto every fourth pile (4, 8, 12, etc.).
Each pile should now contain four cards. Take them up in order, starting with the first.
Now comes the payoff. Spell aloud A-C-E, dealing a card for each letter and turning the last one face up. It will be an ace. Continue with T-W-O, T-H-R-E-E, and so on up through J-A-C-K, Q-U-E-E-N, and K-I-N-G. In each case the last card will have the rank just spelled — and the full count will precisely exhaust the deck.
(From Robert Morrison Abraham, Winter Nights Entertainments, A Book of Pastimes for Everybody, 1932.)
Here’s a foolproof way to get anyone to sleep with you. Ask:
“If she keeps her word,” writes Richard Mark Sainsbury, “she must answer Yes to the second question, whatever she has answered to the first.”
To multiply 1,639,344,262,295,081,967,213,114,754,098,360,655,737,704,918,032,787 by 71, all you have to do is to place another 1 at the beginning and another 7 at the end.
— Samuel Isaac Jones, Mathematical Wrinkles, 1929

The son of William Henry Harrison, John Scott Harrison, served two terms in Congress but spent the rest of his life quietly on his farm in Ohio. At his interment there in 1878 it was discovered that an adjoining grave had recently been robbed, so Harrison’s son and nephew traveled to Cincinnati to seek the missing body.
They visited several medical schools but found nothing, and were about to give up when they noticed a tautened rope leading into chute in the dissecting room of the Ohio Medical College. On turning the windlass they brought up a naked body and discovered to their horror that it was Harrison himself, his body stolen somehow from a guarded brick vault less than 24 hours after his burial.
By a curious further coincidence, Harrison’s son Benjamin himself eventually grew up to be president, making John Scott Harrison the only man in history to be both son and father of a U.S. president. But no one knows who stole his body, how, or how it came to be suspended in that dissecting room.