Puzzles
Wild Kingdom
Fourteen ladders stand in a row. At the foot of each ladder is a monkey; at the top is a banana. Festooning the ladders are an arbitrary number of ropes. Each rope connects a rung on one ladder to a rung on another, but no rung receives more than one rope.
At a signal all 14 monkeys begin climbing. If a monkey encounters a rope it climbs along it to the other end and then continues climbing upward. Show that every monkey gets a banana.
Black and White
Unwound
You have 13 reels of magnetic tape, one empty reel, and a machine that will wind tape from a full reel to an empty one, reversing its direction. You need all 13 tapes reversed on their original reels. Show how this can be done, or prove that it’s impossible.
Tall and Wide
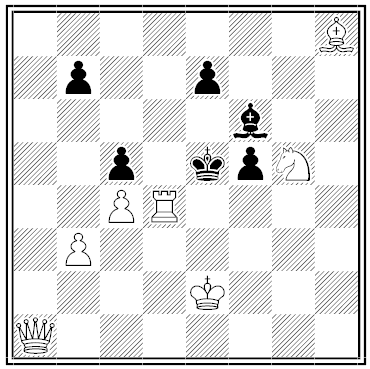
Black and White
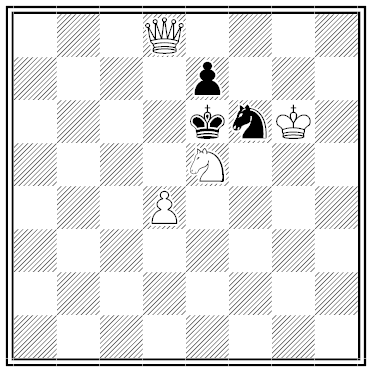
By H. Van Beek, Chemnitzer Wochenschach, 1926 (special prize). White to mate in two moves.
Outpourings
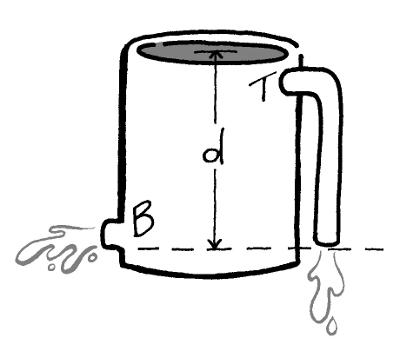
A tank of water has two holes of equal area, one at top and one at bottom. The top one leads to a downspout, so that both holes discharge their water at the same level. Ignoring friction, which hole produces the faster flow of water?
You actually don’t need to know the physics in order to solve this — it yields to an insight.
Substantial

Foaled in 1773, this thoroughbred racehorse bore the unlikely name of Potoooooooo.
How was it pronounced?
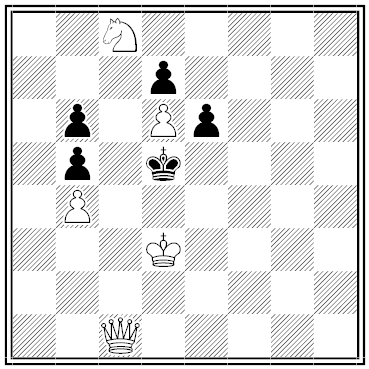
Black and White
Tangled Tale
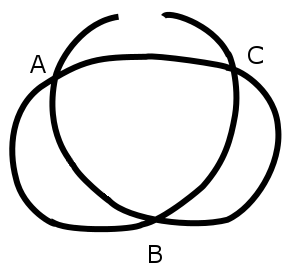
A shoelace is lying on the floor, and I’m too nearsighted to see how the lace crosses itself at points A, B, and C. If I pull on the ends, what’s the probability that it will produce a knot?