You have 100 coins totaling $5.00. They consist of pennies, dimes, and half dollars. How many of each are there?
Puzzles
Five Puzzles
- In a footrace you overtake the person in second place. What position are you now in?
- A clock costs $10 plus half its cost. What does it cost?
- A month begins on Friday and ends on Friday. What month is it?
- What’s the next letter in this sequence? W T N L I T _
- The 22nd and 24th U.S. presidents had the same mother and father but were not brothers. How?
Hand Over Hand

What is the best full house? Suppose you are playing poker and a genie offers to arrange the deal so that you receive the full house of your choice. What hand should you specify?
Till Death …
The law in a certain village requires that any wife who can prove that her husband has been unfaithful must shoot him before sundown on the same day. Every wife reasons perfectly, and all the wives know this. Further, every wife knows instantly when another’s husband has been unfaithful but never whether her own is.
One day the mayor announces that there is at least one unfaithful husband in the village. In fact there are 40 unfaithful husbands, but the wives do not know this. What happens?
Black and White
Small World

Three flying saucers land simultaneously on a spherical planet.
What’s the probability that all land in or on the same hemisphere?
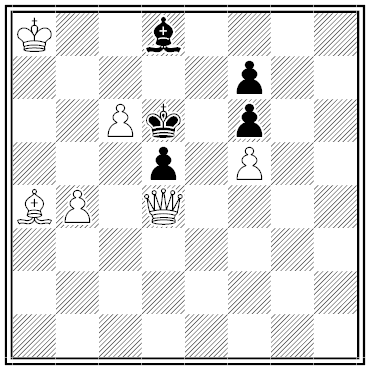
Black and White
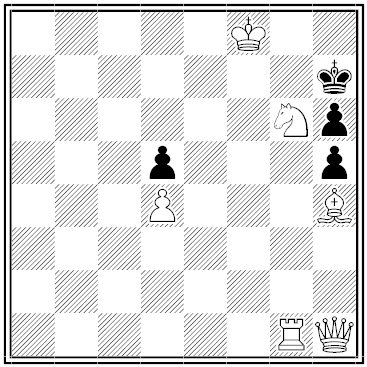
By Joseph Kling, from Chess Euclid, 1849. White to mate with the pawn in three moves.
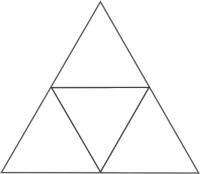
Elbow Room
Five points are located in an equilateral triangle with 10-inch sides (or on its perimeter). What’s the maximum distance between the two closest points?
Hocus Pocus
A magician invites me to do the following:
- Open a brand-new deck of playing cards and place it face down on the table.
- Count off the top 20 cards, turn them face up, and insert them at random into the remainder of the deck.
- Shuffle the deck thoroughly.
- Count off the top 20 cards and hand them to him under the table.
Now, after a period of mysterious activity under the table, he places his pack of 20 cards next to mine of 32. Using the dark arts, he says, he has arranged that both packs now contain the same number of face-up cards. We count, and they do. How has he accomplished this?
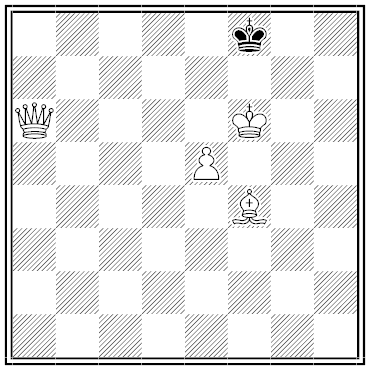
Black and White
From Benjamin Glover Laws, The Two-Move Chess Problem, 1890. White to mate in two moves.