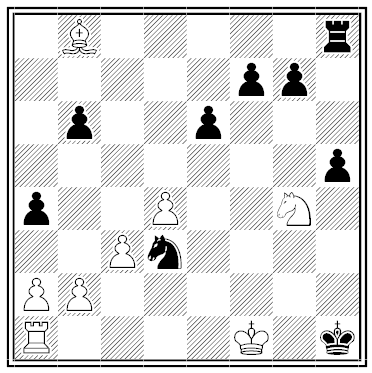
By Sam Loyd. White is kicking himself — he has just completed a move, and realizes that he could have given mate instead. What was his move, and what was the mate?

By Sam Loyd. White is kicking himself — he has just completed a move, and realizes that he could have given mate instead. What was his move, and what was the mate?
In the November 1971 issue of Word Ways, reader Solomon Golomb noted that the following placard appeared in the window of a business establishment “and was considered quite ordinary by all passers-by.” Why?

Babylas, Hilary, and Sosthenes have escaped the tower and divided their treasure into three bags. But now they must cross a river, and the boat can accommodate only two men at a time, or one man and a bag. None will trust another with his bag on the shore, but they agree that a man in the boat can be trusted to drop or retrieve a bag at either shore, as he’ll be too busy to tamper with it. How can they cross the river?

Three robbers, Babylas, Hilary, and Sosthenes, are stealing a treasure chest from the top of an old tower. Unfortunately, they’ve had to destroy their ladder to avoid pursuit, so they’ll have to descend using a crude tackle — a single pulley and a long rope with a basket at each end.
Babylas weighs 170 pounds, Hilary 100 pounds, Sosthenes 80 pounds, and the treasure 60 pounds. If the difference in weight between the two baskets is greater than 20 pounds then the heavier basket will descend too quickly and injure its occupant (though the treasure chest can withstand this). How can the three of them safely escape the tower with the treasure?

Upend Congress and pour its members onto Constitution Avenue. Each member has up to three enemies. Prove that it’s possible to pack the 535 members back into the House and Senate in such a way that none of them has more than one enemy in his chamber. (Enmity is always mutual — I am my enemy’s enemy — and the chambers need not retain their former sizes.)

Ten weary, footsore travelers,
All in a woeful plight,
Sought shelter at a wayside inn
One dark and stormy night.
“Nine beds — no more,” the landlord said,
“Have I to offer you;
To each of eight a single room,
But the ninth must serve for two.”
A din arose. The troubled host
Could only scratch his head,
For of those tired men, not two
Could occupy one bed.
The puzzled host was soon at ease —
He was a clever man —
And so to please his guests devised
This most ingenious plan.
In room marked A, two men were placed,
The third he lodged in B,
The fourth to C was then assigned —
The fifth retired to D.
In E the sixth he tucked away,
In F the seventh man;
The eighth and ninth in G and H,
And then to A he ran,
Wherein the host, as I have said,
Had laid two travelers by;
Then taking one, the tenth and last,
He lodged him safe in I.
Nine single rooms — a room for each —
Were made to serve for ten.
And this it is that puzzles me
And many wiser men.
— S.R. Ford, Ford’s Christian Repository & Home Circle, May 1888

A puzzle from Lewis Carroll’s diary:
The Dodo says that the Hatter tells lies.
The Hatter says that the March Hare tells lies.
The March Hare says that both the Dodo and the Hatter tell lies.
Who is telling the truth?
A riddle by Jonathan Swift:
We are little airy creatures,
All of different voice and features:
One of us in glass is set,
One of us you’ll find in jet,
T’other you may see in tin,
And the fourth a box within;
If the fifth you should pursue,
It can never fly from you.
What are we?