Puzzles
Sudden Death
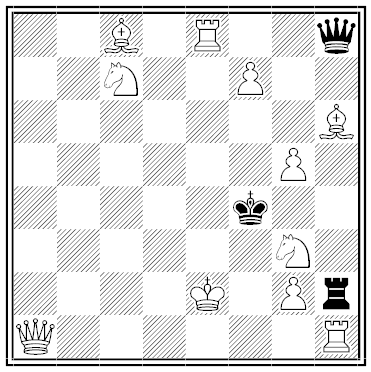
“Paul Morphy IV” submitted this puzzle to the Journal of Recreational Mathematics in 1974. White might capture Black’s queen at this point, but instead can choose to mate on the move. How?
“A Desperate Woman”
A puzzle from Henry Dudeney:
Her eyes were filled with tears, her face was flushed with anger, and her expression was one of indignation at the brutal injury to which she had been subjected.
“You monster of cruelty!” she cried, “I have borne with you too long! The very foundations of my being you have injured. Day by day I have endured your tortures. When first we met your ease and polish attracted me, and when you became my own my friends envied me. Yet see what I have suffered for your sake! You offer every opposition to my advancing myself. Your understanding is far too small for a large soul like mine. My standing in society you have entirely ruined. Had we never met I might have walked in peace. Begone! We part for ever.”
There was a moment’s convulsive breathing, a grinding of teeth, and a quick sigh. It was all over between them. One supreme effort, and she cast it from her —
“‘Whom?’ perhaps the reader will ask. That is the question. I leave you to fill in the blank.”
Fatal Attraction
You have two straight iron bars that look the same. One is a magnet and one is not. How can you tell which is which only by touching them together?
False Fealty
Written in prison by Arthur Connor, a prominent figure in the Irish Rebellion of 1798:
The pomps of Courts and pride of kings
I prize above all earthly things;
I love my country, but the King,
Above all men, his praise I sing.
The Royal banners are displayed,
And may success the standard aid.
I fain would banish far from hence
The “Rights of Man” and “Common Sense.”
Confusion to his odious reign,
That foe to princes, Thomas Paine.
Defeat and ruin seize the cause
Of France, its liberties and laws.
Connor escaped in 1807 and made his way to France, where he became a general in the army. “These two apparently loyal verses, if properly read, bear a very different meaning,” writes Henry Dudeney. “Can you discover it?”
Black and White
A Puzzling Figure

A poser from Jerome S. Meyer’s Puzzle Quiz and Stunt Fun (1956):
“The mark above is the mark of evil. It is the symbol of murder and terror. Millions have died for it and millions have died because of it. Can you tell what it is?”
Sudden Death
Twenty-five basketball teams compete in a knockout tournament — each team is eliminated after its first loss. How many games must be played to establish a winner?
Fated
1. P-K4 P-K4 2. B-B4 P-R4 3. Q-B3 P-R4 4. QxP mate:
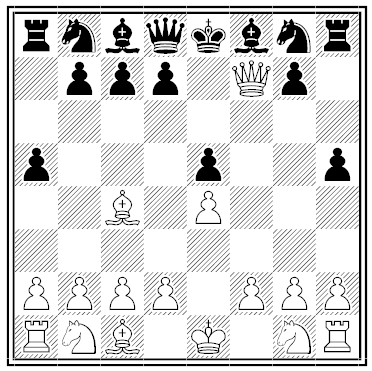
That’s a pretty colorless chess game, but Mike Keith points out that the game score makes an alphametic:
P-K4 + P-K4 + B-B4 + P-R4 + Q-B3 + P-R4 + QxP = IWIN
Replace each letter and symbol (-, x) with a digit, leaving the numbers as they are, to produce a valid equation.
Three Puzzles
1. You are midway through dealing a bridge hand when a nuclear apocalypse destroys civilization. The bridge table is still upright, and your friends are willing to keep playing, but you can’t remember where you left off dealing. How can you finish dealing this hand without having to start over?
2. Can I stand behind you while you stand behind me?
3. My bed is 10 feet from the light switch. But last week I turned off the light, dashed across the room, and was in bed before the room got dark. How did I manage this?