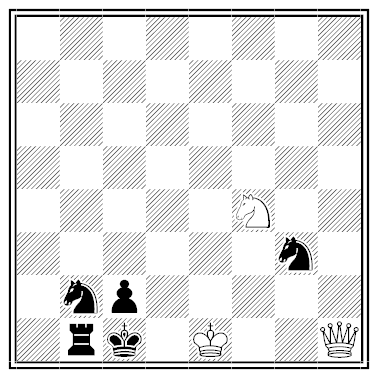
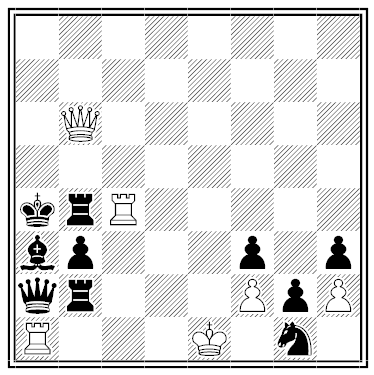
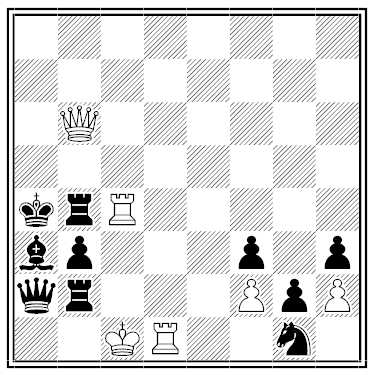
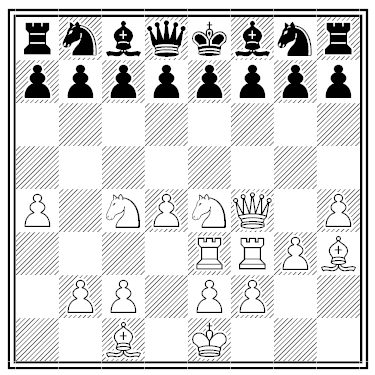
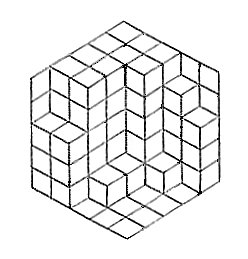
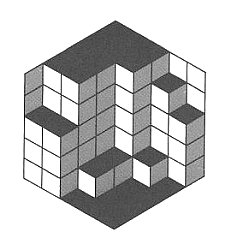
A puzzle from R.M. Abraham, Diversions & Pastimes, 1933:
Michael O’Bleary hired a motor-car at a cost of fifteen dollars to take him to Ballygoogly market and back again in the evening. When he got half-way on his outward journey he met a friend, gave him a lift to the market, and brought him back to the point where he picked him up in the morning. There was a dispute about the payment. How much should Michael charge his passenger for his share of the motor hire?