Many masculine nouns can be converted to feminine with a suffix, as HERO-HEROINE and HOST-HOSTESS.
Name a feminine noun that can be converted to masculine with a suffix.
Many masculine nouns can be converted to feminine with a suffix, as HERO-HEROINE and HOST-HOSTESS.
Name a feminine noun that can be converted to masculine with a suffix.

Every room in my house has an even number of doors.
Prove that the house has an even number of exterior doors.
One train leaves Los Angeles for New York at 60 mph.
At the same time, another train leaves New York for Los Angeles at 40 mph.
What is the distance between them one hour before they meet?
A.A. Bennett offered this puzzle in the American Mathematical Monthly of May 1937:
A car with n (n > 2) passengers of different speeds of mental reaction passes through a tunnel and each passenger acquires unconsciously a smudge of soot upon his forehead. Suppose that each passenger
(1) laughs and continues to laugh as soon as and only so long as he sees a smudge upon the forehead of a fellow passenger;
(2) can see the foreheads of all his fellows;
(3) reasons correctly;
(4) will clean his own forehead when and only when his reasoning forces him to conclude that he has a smudge;
(5) knows that (1), (2), (3), and (4) hold for each of his fellows.
Show that each passenger will eventually wipe his own forehead.
A puzzle by Isaac Asimov:
What word in the English language changes its pronunciation when it is capitalized?
A motorcyclist was sent by the post office to meet a plane at the airport.
The plane landed ahead of schedule, and its mail was taken toward the post office by horse. After half an hour the horseman met the motorcyclist on the road and gave him the mail.
The motorcyclist returned to the post office 20 minutes earlier than he was expected.
How many minutes early did the plane land?
A poser from 1821:
Mathematicians affirm that of all bodies contained under the same superficies, a sphere is the most capacious: But they have never considered the amazing capaciousness of a body, the name of which is now required, of which it may be truly affirmed, that supposing its greatest length 9 inches, greatest breadth 4 inches, and greatest depth 3 inches, yet under these dimensions it contains a solid foot?
What is this body?
A problem posed by Harry Houdini: Given a piece of cardboard measuring 4″ × 2.5″, cut it so that a person can pass completely through it without tearing it.
Can it be done?
From the American journal Scripta Mathematica:
An elementary school teacher in New York state had her purse stolen. The thief had to be Lilian, Judy, David, Theo, or Margaret. When questioned, each child made three statements:
Lilian:
(1) I didn’t take the purse.
(2) I have never in my life stolen anything.
(3) Theo did it.
Judy:
(4) I didn’t take the purse.
(5) My daddy is rich enough, and I have a purse of my own.
(6) Margaret knows who did it.
David:
(7) I didn’t take the purse.
(8) I didn’t know Margaret before I enrolled in this school.
(9) Theo did it.
Theo:
(10) I am not guilty.
(11) Margaret did it.
(12) Lillian is lying when she says I stole the purse.
Margaret:
(13) I didn’t take the teacher’s purse.
(14) Judy is guilty.
(15) David can vouch for me because he has known me since I was born.
Later, each child admitted that two of his statements were true and one was false. Assuming this is true, who stole the purse?
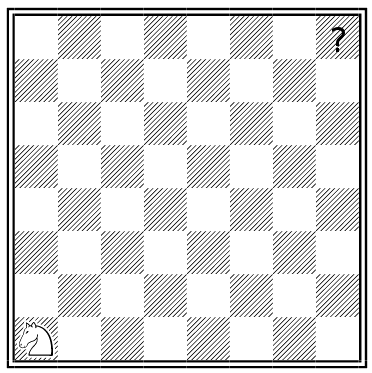
Is it possible to move the knight from a1 to h8, visiting every square of the chessboard once?