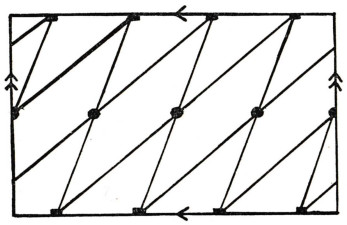
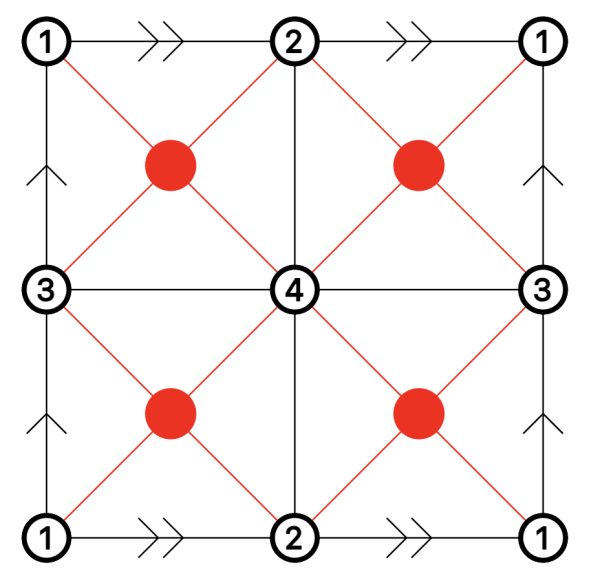
During the middle ages there existed a monastery, in which lived twenty-four monks, presided over by a blind abbot. The cells of the monastery were planned as shown in the accompanying figure, passages being arranged along two sides of each of the outer cells and all round the inner cell, in which the abbot took up his quarters. Three monks were allotted to each cell, making, of course, nine monks in each row of cells. The abbot, being lazy as well as blind, was very remiss in making his rounds, but provided he could count nine heads on each side of the monastery he retired into his own cloister, contented and satisfied that the monks were all within the building, and that no outsiders were keeping them company. The monks, however, taking advantage of their abbot’s blindness and remissness, conspired to deceive him, a portion of their number sometimes going out and at other times receiving friends in their cells. They accomplished their deception, and it never happened that strangers were admitted when monks were out, yet there never were more nor less than nine persons upon each side of the building. Their first deception consisted in four of their number going out, upon which four monks took possession of each of the cells numbered 1, 3, 6, and 8, one monk only being left in each of the other cells; nine monks being thus on each side of the building. Upon returning, the four monks brought in four friends, when it was necessary to arrange the twenty-eight persons, two in each of the cells 1, 3, 6, and 8, and five in each of the others; still nine heads only were to be counted in either row. Emboldened by success, eight outsiders were introduced, and the thirty-two persons now were arranged, one only in each of the cells 1, 3, 6, and 8, but seven in each of the other cells; again, according to the abbot’s system of counting, all was well. In the next endeavour, the strangers all went away and took six monks with them, leaving but eighteen at home to represent twenty-four; these eighteen placed themselves five in each of the cells 1 and 8 and four in each of the cells 3 and 6; the remaining cells were empty, but the cells on each side of the building still contained nine monks. On returning, the six truants each brought two friends to pass the night, and the thirty-six retired to rest, nine in each of the cells 2, 4, 5, and 7; the remainder were empty, and the abbot was quite satisfied that the monks were alone in the monastery.
— Cassell’s Book of In-Door Amusements, Card Games and Fireside Fun, 1882