- Which is worth more, a pound of $10 gold pieces or half a pound of $20 gold pieces?
- A kazoo costs $1 plus half its price. How much does it cost?
- On its March 1961 cover, MAD Magazine pointed out that 1961 was the first “upside-up” year — the first year that reads the same upside down — since 1881. What will be the next such year?
Puzzles
The Stopped Clock
Andrea’s only timepiece is a clock that’s fixed to the wall. One day she forgets to wind it and it stops.
She travels across town to have dinner with a friend whose own clock is always correct. When she returns home, she makes a simple calculation and sets her own clock accurately.
How does she manage this without knowing the travel time between her house and her friend’s?
Dilemma
You’re given a choice between two gifts: $5 and $1,000. You can choose either, but a bystander will give you $1 million if you choose irrationally. Can you do it?
See also Kavka’s Toxin Puzzle.
Find the Theme, Part 2
What do these words have in common?
- ALMOND
- INCOME
- CANDID
- MEMORIAL
- CONCORDE
- GANYMEDE
- MANDARIN
- MAINLAND
Hell’s Bells
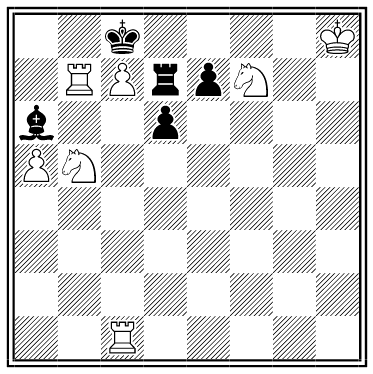
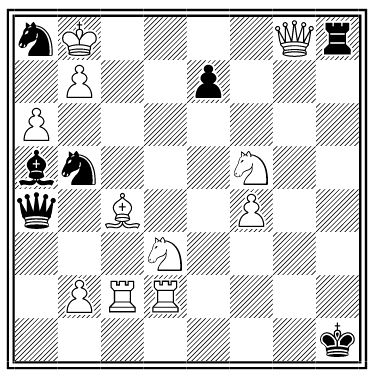
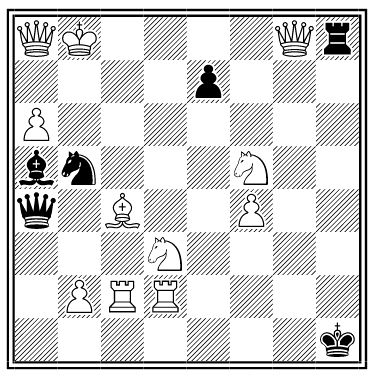
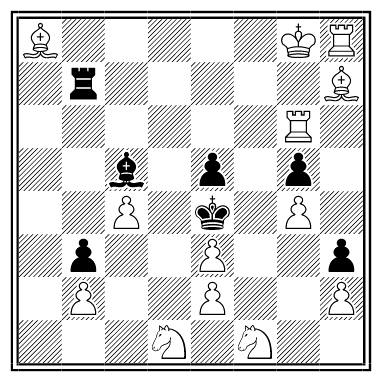
A Christmas puzzle by J.C.J. Wainwright, from the American Chess Bulletin, December 1917.
White to mate in one move.
Retail Trouble
A woman visits a jewelry store and buys a ring for $100.
The next day she returns and asks to exchange it for another. She picks out one worth $200, thanks the jeweler and turns to go.
“Wait, miss,” he says. “That’s a $200 ring.”
“Yes,” she says. “I paid you $100 yesterday, and I’ve just given you a ring worth $100.”
And she trips lightly out of the store.
The Corpse Groom
Dispatch
Find the Theme, Part 1
What do these words have in common?
- BEANED
- DOTTED
- GRANTED
- HERBAL
- HOMERED
- JACKAL
- LEEWARD
- ROYAL
- PATRON
- VICTIM
- VICTORIAN