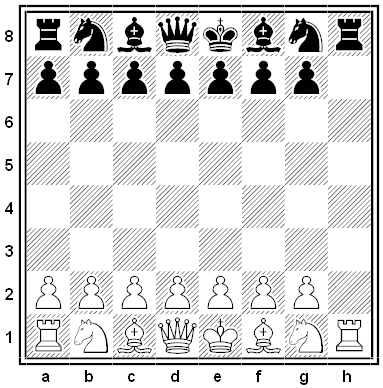
By Éric Angelini. A regular chess game reached this position after Black’s fifth move. Four pieces have moved. Which ones?

By Éric Angelini. A regular chess game reached this position after Black’s fifth move. Four pieces have moved. Which ones?
There are 12 people in a room. Some always tell the truth, and the rest always lie.
#1 says, “None of us is honest.”
#2 says, “There is not more than 1 honest person here.”
#3 says, “There are not more than 2 honest people here.”
#4 says, “There are not more than 3 honest people here.”
#5 says, “There are not more than 4 honest people here.”
#6 says, “There are not more than 5 honest people here.”
#7 says, “There are not more than 6 honest people here.”
#8 says, “There are not more than 7 honest people here.”
#9 says, “There are not more than 8 honest people here.”
#10 says, “There are not more than 9 honest people here.”
#11 says, “There are not more than 10 honest people here.”
#12 says, “There are not more than 11 honest people here.”
How many honest people are in the room?

A puzzle by Henry Dudeney:
‘There’s a mouse in one of these barrels,’ said the dog.
‘Which barrel?’ asked the cat.
‘Why, the five-hundredth barrel.’
‘What do you mean by the five-hundredth? There are only five barrels in all.’
‘It’s the five-hundredth if you count backwards and forwards in this way.’
And the dog explained that you count like this:
1 2 3 4 5 9 8 7 6 10 11 12 13So that the seventh barrel would be the one marked 3 and the twelfth barrel the one numbered 4.
‘That will take some time,’ said the cat, and she began a laborious count. Several times she made a slip, and had to begin again.
‘Rats!’ exclaimed the dog. ‘Hurry up or you will be too late!’
‘Confound you! You’ve put me out again, and I must make a fresh start.’
Meanwhile the mouse, overhearing the conversation, was working madly at enlarging a hole, and just succeeded in escaping as the cat leapt into the correct barrel.
‘I knew you would lose it,’ said the dog. ‘Your education has been sadly neglected. A certain amount of arithmetic is necessary to every cat, as it is to every dog. Bless me! Even some snakes are adders!’
Now, which was the five-hundredth barrel? Can you find a quick way of arriving at the answer without making the actual count?
A problem by Russian mathematician Vyacheslav Proizvolov:
At a party each girl danced with three boys, and each boy danced with three girls. Prove that the number of girls at the party was equal to the number of boys.
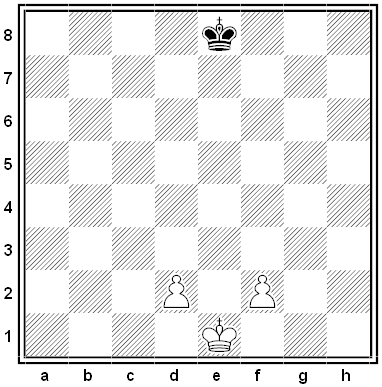
A problem by Raymond Smullyan. The diagram above shows the final position in a chess game in which nothing has moved from a white square to a black one or vice versa. One piece has been omitted from the diagram. What color is the square that it stands on?
A puzzle by Henry Dudeney:
When visiting with a friend one of our hospitals for wounded soldiers, I was informed that exactly two-thirds of the men had lost an eye, three-fourths had lost an arm, and four-fifths had lost a leg. ‘Then,’ I remarked to my friend, ‘it follows that at least twenty-six of the men must have lost all three — an eye, an arm, and a leg.’ That being so, can you say exactly how many men were in the hospital? It is a very simple calculation, but I have no doubt it will perplex a good many readers.
A man goes into a 7-11 store, buys four items, and notices that the bill totals $7.11. Even more interestingly, the product of the four prices is 7.11. What are the prices?
The answer is $1.20, $1.25, $1.50, and $3.16. There’s no thunderbolt insight to find; the problem yields to patient consideration.
Who came up with it? The most common credit I’ve seen is Doug Brumbaugh of the University of Central Florida. I found it in Crux Mathematicorum; see problem M203 in the September 2006 issue for Richard K. Guy’s solution.
A puzzle by V. Dubrovsky, from Quantum, January-February 1992:
In a certain planetary system, no two planets are separated by the same distance. On each planet sits an astronomer who observes the planet closest to hers. Prove that if the total number of planets is odd, there must be a planet that no one is observing.
Arthur and Robert are identical twins. One always lies, and the other always tells the truth, but you don’t know which is the liar. One day you meet one of them and want to find out whether it’s Arthur or Robert. But you can ask only one yes/no question, and the question can’t contain more than three words. What question will do? Alternatively, suppose you want to find out whether it’s Arthur or Robert who’s truthful. What three-word yes/no question will reveal the answer?