
A quickie from Peter Winkler’s Mathematical Puzzles, 2021: Can West Virginia be inscribed in a square? That is, is it possible to draw some square each of whose four sides is tangent to this shape?

A quickie from Peter Winkler’s Mathematical Puzzles, 2021: Can West Virginia be inscribed in a square? That is, is it possible to draw some square each of whose four sides is tangent to this shape?
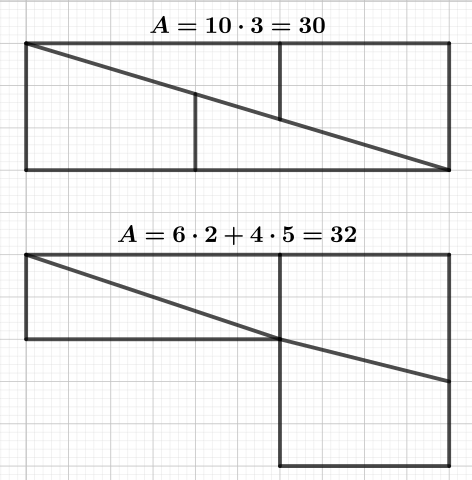
William Hooper published the oddity in 1774. The rectangle at the top measures 10 units by 3, giving an area of 30. But its dissected pieces seem to produce two other rectangles, with areas 12 and 20. Where did the two extra units come from?
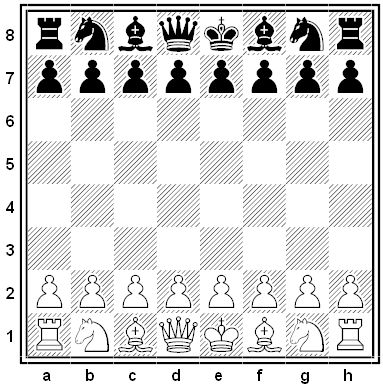
A puzzle by Henry Dudeney:
A chessboard was on the table with the pieces all set up for a game. So I asked Dr. Bates to play a game with the Major on these conditions: Whatever move Bates made throughout, with the white pieces, the Major must exactly imitate with the black, and Bates must give checkmate on the fourth move. As an experiment, Bates started off with 1. e4, and Rackford replied with 1. e5. Then Bates played 2. Qh5, and the Major had to reply with 2. Qh4. This gave me a good opportunity to explain that White cannot now play 3. QxQ, because it would be impossible for Black then to imitate the move. Neither could he play 3. Qxf7+, because Black cannot do the same thing, as he would have to get out of check. White must always make a move that Black can copy, until the checkmate is actually given on the fourth move.
“This puzzle caused great interest, and it was some time before somebody (I think it was Strangways) hit on a solution.”
The name of one chemical element appears as an unbroken string in the names of four other elements. What is the element, and what are the four?
A problem from the Leningrad Mathematical Olympiad: You have 32 stones, each of a different weight. How can you find the two heaviest in 35 weighings with an equal-arm balance?
Express 1,000,000 as the product of two numbers, neither of which contains any zeroes.
A problem from the Leningrad Mathematical Olympiad: A and B take turns removing matches from a pile. The pile starts with 500 matches, A goes first, and the player who takes the last match wins. The catch is that the quantity that each player withdraws on a given turn must be a power of 2. Does either player have a winning strategy?
From John Winter Jones’ Riddles, Charades, and Conundrums, 1822:
What is that which a coach always goes with, cannot go without, and yet is of no use to the coach?
Noise.
***
From Routledge’s Every Boy’s Annual, 1864:
Why is O the noisiest of the vowels?
Because all the rest are inaudible (in audible).
***
From Mark Bryant’s Riddles: Ancient & Modern, 1983:
What is big at the bottom, little at the top, and has ears?
A mountain (it has mountaineers!).