Puzzles
Black and White
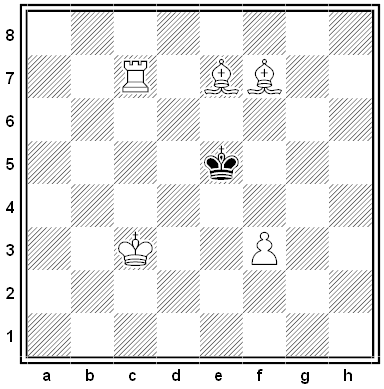
A problem “for juvenile solvers” by Edith Baird, from Womanhood, May 1899. White to mate in two moves.
A Confusing Country
Philosopher Nelson Goodman published this puzzle anonymously in the Boston Post in 1931, at age 24. He later called it “by far the most popular and widely circulated of all my writings.”
All the men of a certain country are either nobles or hunters, and no one is both a noble and a hunter. The male inhabitants are so nearly alike that it is difficult to tell them apart, but there is one difference: nobles never lie, and hunters never tell the truth.
Three of the men meet one day and Ahmed, the first, says something. He says either, ‘I am a noble’, or ‘I am a hunter.’ (We don’t know yet which he said.)
Ali, the second man, heard what Ahmed said, and in reply to a query, answered, ‘Ahmed said, “I am a hunter”.’ Then Ali went on to say, ‘Azab is a hunter.’
Azab was the third man. He said, ‘Ahmed is a noble.’
Now the problem is, which is each? How do you know?
Bright Idea
A jailer will send each of a group of n prisoners alone into a certain room. Each prisoner will visit the room infinitely often, but the order of the visits will be determined arbitrarily by the jailer. The prisoners can confer in advance, but once the visits have commenced they can communicate with one another only by means of a light in the room, which they can turn on or off. How can they ensure that some prisoner will eventually be able to determine that everyone has visited the room?
Black and White
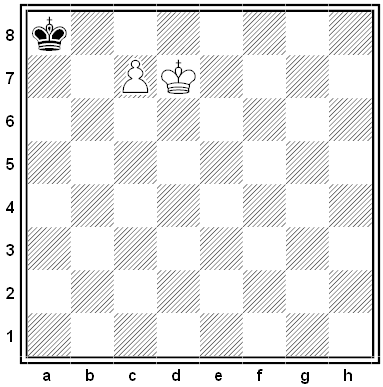
A simple but pretty “lightweight” problem by R. Steinweg. White to mate in three moves.
“Piccadilly Underground Station”
This unusual puzzle by G.A. Roberts appeared in the January 1941 issue of Eureka, the journal of recreational mathematics published at Cambridge University. It concerns the Piccadilly Circus station of the London Underground, which lies on the Piccadilly line between Green Park and Leicester Square and on the Bakerloo line between Charing Cross and Oxford Circus.
At a given time there are on the platform, escalators and subways, and in the trains, 128 people, all of whom travel by train, and none of whom return immediately by the way they have come.
Those who have come via Leicester Square are equal in number to those who are about to travel via Leicester Square.
The number of people who arrived by Bakerloo Line is equal to the number who intend to leave by the Piccadilly Line.
The number of people who are travelling from the street to stations on the Piccadilly Line is equal to six-thirteenths of the number who change from the Piccadilly Line to the Bakerloo.
The number who arrive from Green Park and then change to the Bakerloo is equal to the number who are about to travel via Green Park.
The number who are travelling from the street to the Bakerloo is equal to four times the number who arrive in Piccadilly trains but do not use the Bakerloo Line, and of these, twice as many come from Green Park as from Leicester Square.
By how many does the number of people who use the Bakerloo Line exceed that of those who do not?
Black and White
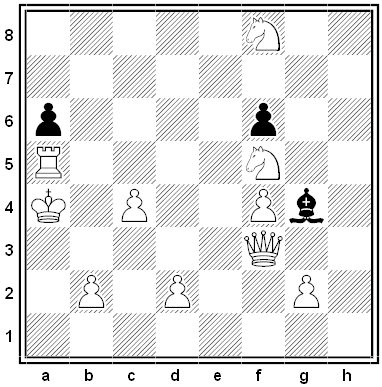
A chess problem from the Jamaica Gleaner. There’s no black king on this board. Find a place for him such that White can mate him in two moves.
Route Cause
A problem submitted by France and shortlisted for the 17th International Mathematical Olympiad, Burgas-Sofia, Bulgaria, 1975:
A lake has six ports. Is it possible to arrange a series of routes that satisfy the following conditions?
- Each route must include exactly three ports.
- No two routes may contain the same three ports.
- Any tourist who wants to visit two different arbitrary ports has a choice of exactly two routes.
Lucky Seven
A puzzle from the National Security Agency’s Puzzle Periodical, posed by NSA mathematician David G. in March 2017:
Each die of a pair of non-identical dice has six faces, but some numbers are missing, others are duplicated, and some faces may have more than six spots.
The dice can roll every number from 2 to 12.
What is the largest possible probability of rolling a 7?
Black and White
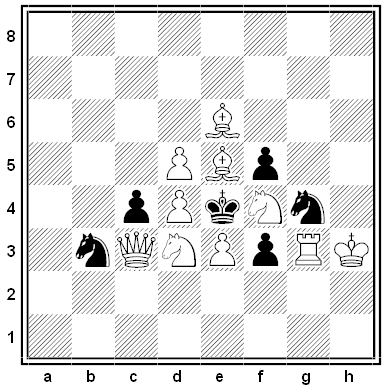
“The Pyramid,” by A.C. White, from the British Chess Magazine. White to mate in two moves.