What’s the next number in this sequence?
1, 2, 2, 3, 3, 4, 4, 4, 5, 5, 5, 6, 6, 6, 6, 7, 7, 7, 7, 8, 8, 8, 8, 9, 9, 9, 9, …
What’s the next number in this sequence?
1, 2, 2, 3, 3, 4, 4, 4, 5, 5, 5, 6, 6, 6, 6, 7, 7, 7, 7, 8, 8, 8, 8, 9, 9, 9, 9, …
From a Russian puzzle collection:
Is it possible to bake a cake that can be divided into four parts by a single straight cut?
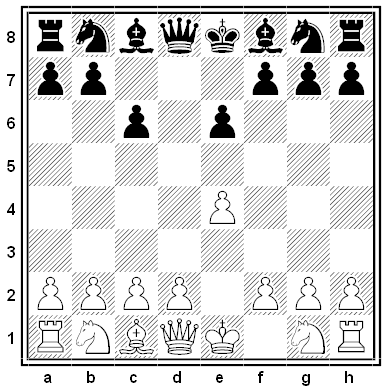
Tibor Orbán offered this puzzle in Die Schwalbe in 1976. The position above can be reached in exactly 3 moves in several ways — for example:
1. e4 c6 2. Bb5 e6 3. Bxc6 dxc6
1. e4 e6 2. Bc4 c6 3. Bxe6 dxe6
How can it be reached in exactly 4 moves?
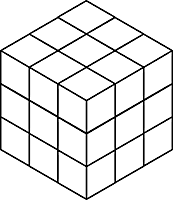
A mouse wants to eat his way through a 3 × 3 × 3 cube of cheese, starting in one of the corners and tunneling through all 27 1 × 1 × 1 sub-cubes, visiting each once. Can he arrange his route so that he finishes at the center of the cube? Assume that he always moves between orthogonally adjacent cubes, traveling through walls but not through edges or corners.
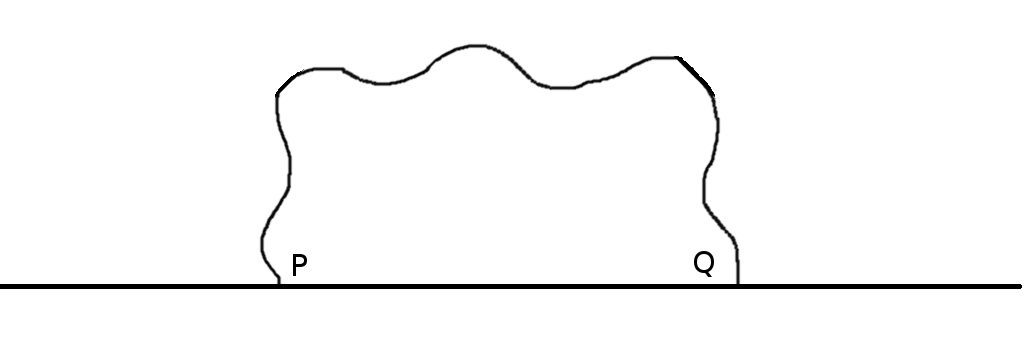
University of Illinois mathematician John Wetzel called this one of his favorite problems in geometry. Call a plane arc special if it has length 1 and lies on one side of the line through its end points. Prove that any special arc can be contained in an isosceles right triangle of hypotenuse 1.

Here are six new lateral thinking puzzles — play along with us as we try to untangle some perplexing situations using yes-or-no questions.
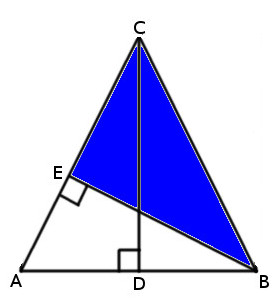
In isosceles triangle ABC, CD = AB and BE is perpendicular to AC. Show that CEB is a 3-4-5 right triangle.
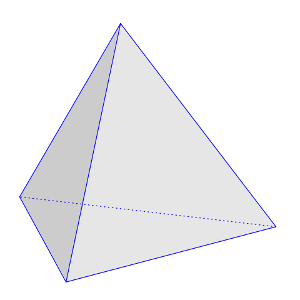
A puzzle from Knowledge, Feb. 1, 1887:
“Show how to cut a regular tetrahedron (equilateral triangular pyramid) so that the face cut shall be a square.”
Let’s play a game. You name an integer from 1 to 10. Then we’ll take turns adding an integer from 1 to 10 to the number our opponent has just named, giving the resulting sum as our answer. Whoever reaches 100 first is the winner.
You go first. What number should you choose?