In Anthony Boucher’s short story “QL 696.C9,” a librarian is found dead at her desk. She has been shot, and apparently spent her last moments typing the Library of Congress card catalog number that gives the story its title. The killer evidently saw nothing incriminating in this and so left it alone. The investigators have narrowed the list of suspects to junior librarian Stella Swift, children’s librarian Cora Jarvis, library patron James Stickney, and high school teacher Norbert Utter. Who did it?
Puzzles
A Mating Machine
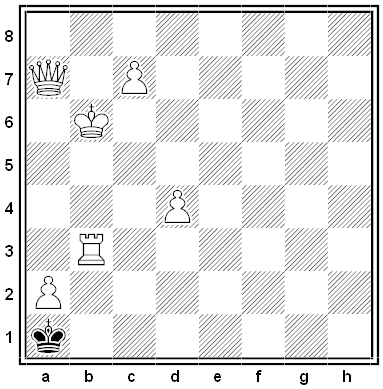
A “no-brainer” by Harvard mathematician (and chess master) Noam Elkies. White has choices as to how to play, but no matter how he proceeds, he’ll wind up mating Black on the seventh move.
(From Tim Krabbé’s chess diary.)
Black and White
Coverup
A problem from the British Columbia Colleges Senior High School Contest for 2000:
If I place a 6 cm × 6 cm square on a triangle, I can cover up to 60% of the triangle. If I place the triangle on the square, I can cover up to 2/3 of the square. What is the area, in cm2, of the triangle?
(a) 22 4/5
(b) 24
(c) 36
(d) 40
(e) 60
Black and White
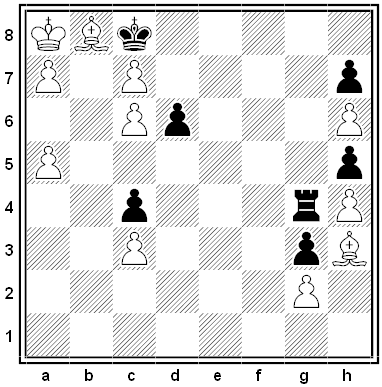
“We now give what is acknowledged to be the finest two-move problem extant,” wrote J.H. Blackburne in the Strand in 1908. “It is by the American expert, W.A. Shinkman, and is also claimed by G.E. Carpenter, a fellow countryman of his. Here we have not only a difficult key-move, but also beauty of theme and artistic construction, the three essential qualities necessary to a perfect problem.”
White to mate in two moves.
The Fifth Element
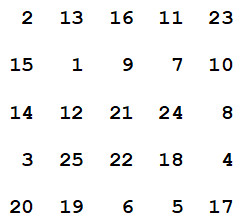
Charles W. Trigg offered this puzzle in the Fall 1977 issue of Pi Mu Epsilon Journal (PDF):
This square array contains the first 25 positive integers. Choose five, no two from the same row or column, so that the largest of the five elements is as small as possible, and justify your choice.
The Impossible Puzzle
Dutch mathematician Hans Freudenthal proposed this puzzle in 1969 — at first it appears impossible because so little information is given.
X and Y are two different whole numbers greater than 1. Y is greater than X, and their sum is no greater than 100. S and P are two logicians; S knows the sum X + Y, and P knows the product X × Y. S and P both reason perfectly, and both know everything I’ve just told you.
- S says, “P does not know X and Y.”
- P says, “Now I know X and Y.”
- S says, “Now I also know X and Y.”
What are X and Y?
Black and White
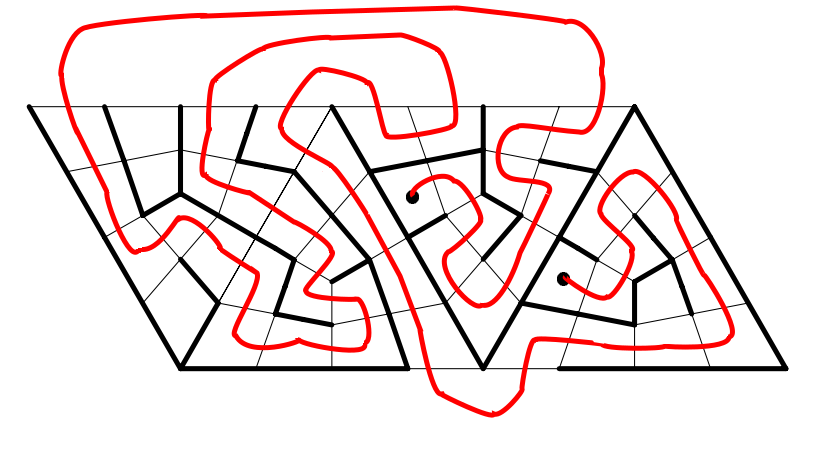
Four-Sided Story

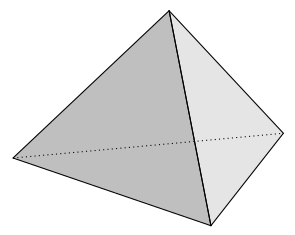
University of Ljubljana electrical engineer Izidor Hafner devised this maze on a tetrahedron, presented as an unfolded plane plan. Can you find a path from one dot to the other? To do so you’ll have to fold up the figure in your head:
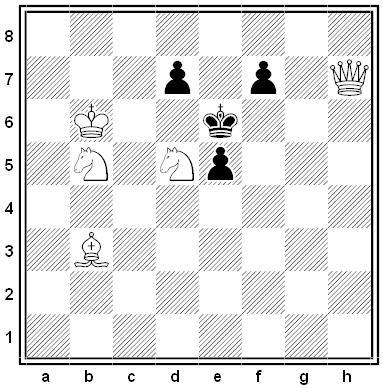
Black and White
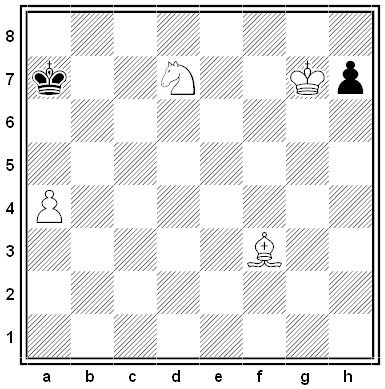
By Augusto Piccinini. Imagine that the board is a vertical cylinder, that is, that the a-file and the h-file are joined so that pieces can move across the border. How can White mate Black in two moves?