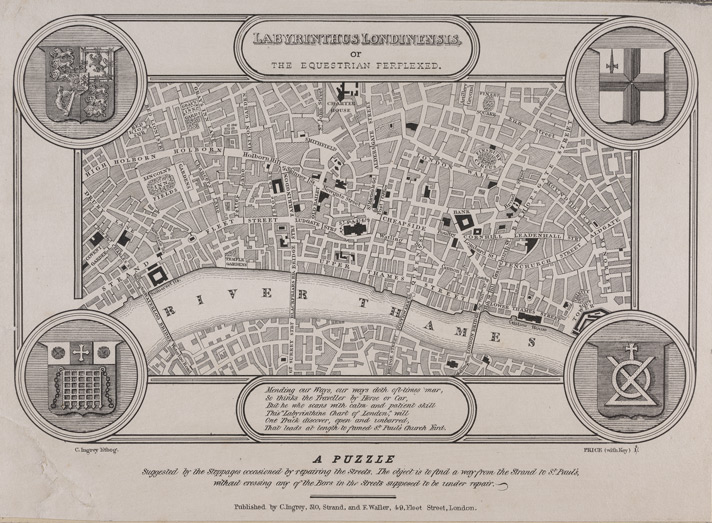
Fed up with endless traffic detours in 1830, London printer Charles Ingrey published a pointed puzzle, Labyrinthus Londoninensis, or The Equestrian Perplexed.
“The object is to find a way from the Strand [lower left] to St. Paul’s [center], without crossing any of the Bars in the Streets supposed to be under repair.”
Mending our Ways, our ways doth oft-times mar,
So thinks the Traveller by Horse or Car,
But he who scans with calm and patient skill
This ‘Labyrinthine Chart of London’, will
One Track discover, open and unbarred,
That leads at length to famed St. Pauls Church Yard.
The image above is a bit too small to navigate, but the British Library has an interactive zoomable version (requires Flash).
I don’t have the solution, but The Court Journal of Dec. 14, 1833, hints that “the farthest way round is the nearest way home.”
07/06/2022 UPDATE: A solution! (Thanks, Paul.)