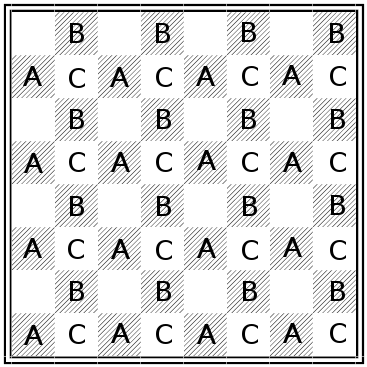
As usual in chess, let the bottom left-hand square be black. If we number the rows from the bottom and the columns from the left, then every black square is either in an odd-numbered row or an even-numbered column. That is, all the black squares can be found in rows 1, 3, 5, 7 and columns 2, 4, 6, 8.
Let the black squares in rows 1, 3, 5, 7 be marked A and the black squares in columns 2, 4, 6, 8 be marked B, and let the white squares in rows 1, 3, 5, 7 be marked C. Leave the rest of the white squares unmarked. Finally, let a, b, c denote the total number of pieces that sit on squares marked A, B, C, respectively. We want to show that a + b is an even number.
Each row contains an odd number of pieces. That means that a + c, the total number of pieces in rows 1, 3, 5, 7, is the sum of four odd numbers, which means it’s even. Likewise, b + c, the total number of pieces in columns 2, 4, 6, 8, is also the sum of four odd numbers and thus even. So
(a + c) + (b + c) = a + b + 2c
is even, and this implies that a + b is even.
(From V. Proizvolov, “Problems Teaching Us How to Think,” Quantum, January-February 2002, via Ross Honsberger’s Mathematical Delights, 2004.)