
In a special football game, a team scores 7 points for a touchdown and 3 points for a field goal. What’s the largest mathematically unreachable number of points that a team can score (in an infinitely long game)?

In a special football game, a team scores 7 points for a touchdown and 3 points for a field goal. What’s the largest mathematically unreachable number of points that a team can score (in an infinitely long game)?

A problem by Atlantic College mathematician Paul Belcher:
Anna and Bert invite n other couples to a dinner party. Before the meal begins, some people shake hands. No one shakes hands with their own partner, no one shakes hands with themselves, and no two people shake hands with each other more than once. Afterward, Anna asks all the other 2n + 1 people how many times they shook hands, and she gets a different answer from each of them. How many times did Anna shake hands?
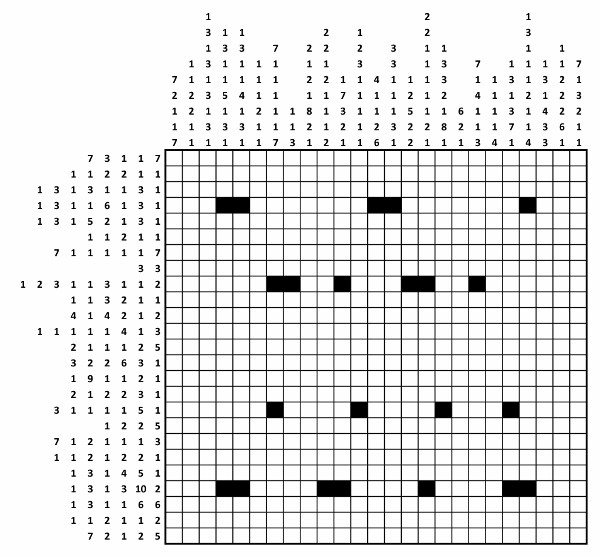
Here’s a unique challenge for the holidays — one of the United Kingdom’s intelligence agencies, GCHQ, is distributing the puzzle above on its Christmas card this year. (See GCHQ’s website for details and a high-resolution grid.)
The puzzle is a nonogram: Each row and column bears a string of numbers that indicates the lengths of consecutive runs of black squares that will appear there when the grid has been completed. For example, “3 3” in the eighth row means that in the finished puzzle two shaded sections of 3 squares each will appear somewhere along its length. Some squares in the grid have already been shaded to get you started.
“By solving this first puzzle players will create an image that leads to a series of increasingly complex challenges,” notes the agency. “Once all stages have been unlocked and completed successfully, players are invited to submit their answer via a given GCHQ email address by 31 January 2016. The winner will then be drawn from all the successful entries and notified soon after.” The agency invites players to make a donation to the U.K.’s National Society for the Prevention of Cruelty to Children if they’ve enjoyed the puzzle.
(Thanks, Alex.)
02/08/2016 UPDATE: The answers have now been revealed — see the link at the bottom of this post.
A problem from the 2004 Harvard-MIT Math Tournament:
Zach chooses five numbers from the set {1, 2, 3, 4, 5, 6, 7} and tells their product to Claudia. She finds that this is not enough information to tell whether the sum of Zach’s numbers is even or odd. What is the product that Zach tells Claudia?
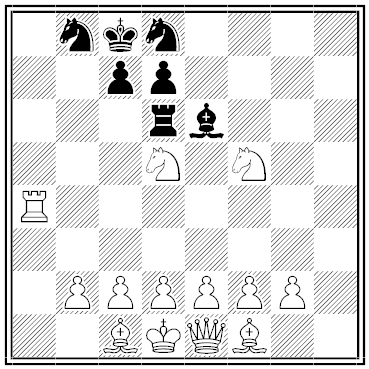
White to play and mate on his first move.
(Don’t spend too much time on this — it’s a bit of a trick.)
In the December 2012 issue of 1 Across magazine, longtime crossword composer John Graham included a special instruction above one of his puzzles:
“I have 18dn of the 19; no 27, just 13 15; no 2 or 6 or 1dn 26 yet — plenty of 10, though I wouldn’t have chosen the timing.”
Solvers discovered that 18 down was CANCER and 19 across was OESOPHAGUS. The full message read:
“I have CANCER of the OESOPHAGUS; no CHEMOTHERAPY, just PALLIATIVE CARE; no NARCOTIC or STENT or MACMILLAN NURSE yet — plenty of MERRIMENT, though I wouldn’t have chosen the timing.”
The puzzle was reprinted as cryptic crossword No. 25,842 in the Guardian the following month.
“It seemed the natural thing to do somehow,” Graham said. “It just seemed right.” He died in November 2013, and the Guardian published a tribute crossword to remember him.
(Thanks, Anthony.)
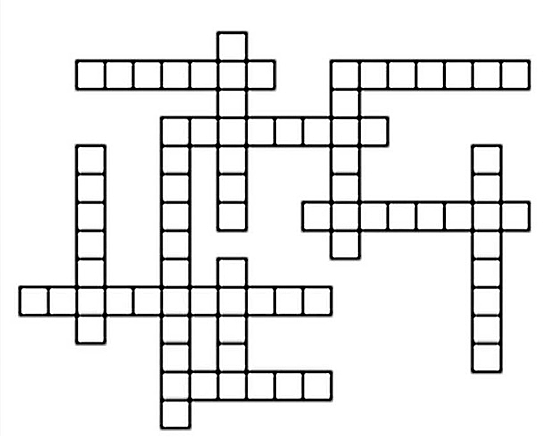
Here’s a unique crossword puzzle by Lee Sallows. There are no clues — instead, each of the 12 entries must take the form [NUMBER](space)[LETTER](S), like so:
EIGHT BS
NINETEEN XS
ONE J
And so on. Can you complete the puzzle so that the finished grid presents an inventory of its own contents?
(A couple observations to get you started: Because the puzzle contains 12 entries, the solution will use only 12 letters. And one useful place to start is the shortest “down” entry, which is too short to be plural — it must be “ONE [LETTER]”.)

Your tedious nephews, Kerry and Kelly, are not honest, but they’re orderly. One of them lies Mondays, Tuesdays, and Wednesdays, and tells the truth on other days, and the other lies on Thursdays, Fridays, and Saturdays, and tells the truth on other days. At noon, they have the following conversation:
Kerry: I lie on Saturdays.
Kelly: I will lie tomorrow.
Kerry: I lie on Sundays.
On which day of the week does this conversation take place?

A baseball poser from Clark Kinnaird’s 1946 Encyclopedia of Puzzles and Pastimes:
“In a spring training game with the Dodgers at bat, the first man up hit a triple, the second man hit a double, the third man hit a double, the fourth man hit a single, the fifth man hit a single, the sixth man hit a single. Yet the Dodgers did not score a run in that inning. How could this have happened? These were the only men who went to bat in the inning.”