Puzzles
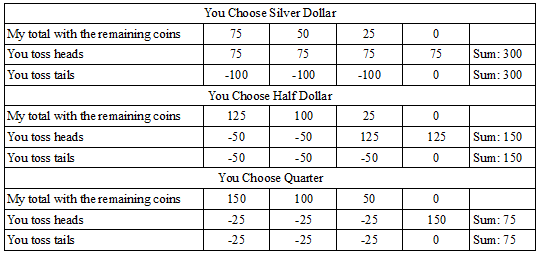
Three Coins
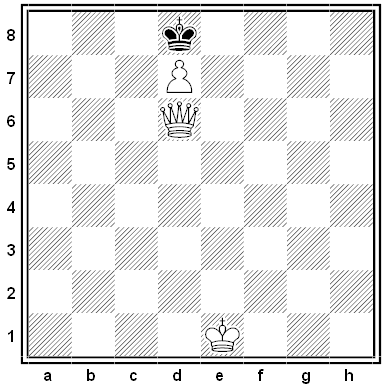
Three coins are lying on a table: a quarter, a half dollar, and a silver dollar. You claim one coin, I’ll claim the other two, and then we’ll toss all three. A coin that lands tails counts zero, and a coin that lands heads wins its value (in cents, 25, 50, or 100) for its owner. Whichever of us has the larger score wins all three coins. If all three coins land tails then we toss again.
Which coin should you claim to make the game fair — that is, so that each of us has an expected win of zero?
Digit Spans
A puzzle by Anatoly Savin: Using each of the digits 1, 2, 3, and 4 twice, write out an eight-digit number in which there is one digit between the 1s, two digits between the 2s, three digits between the 3s, and four digits between the 4s.
The Jeweler’s Observation
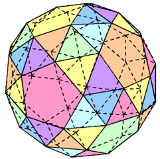
Prove that every convex polyhedron has at least two faces with the same number of sides.
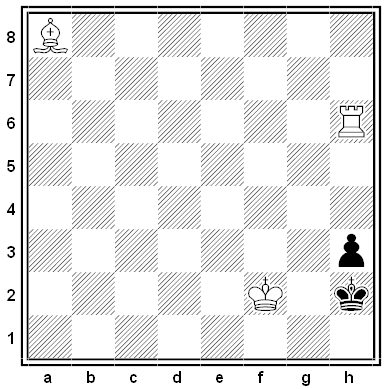
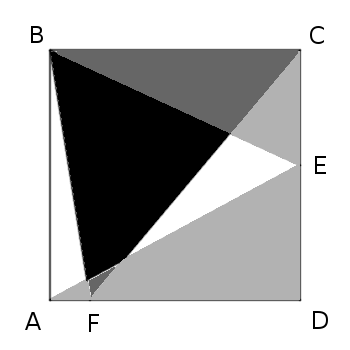
Black and White
Ride Sharing

You and I have to travel from Startville to Endville, but we have only one bicycle between us. So we decide to leapfrog: We’ll leave Startville at the same time, you walking and I riding. I’ll ride for 1 mile, and then I’ll leave the bicycle at the side of the road and continue on foot. When you reach the bike you’ll ride it for 1 mile, passing me at some point, then leave the bike and continue walking. And so on — we’ll continue in this way until we’ve both reached the destination.
Will this save any time? You say yes: Each of us is riding for part of the distance, and riding is faster than walking, so using the bike must increase our average speed.
I say no: One or the other of us is always walking; ultimately every inch of the distance between Startville and Endville is traversed by someone on foot. So the total time is unchanged — leapfrogging with the bike is no better than walking the whole distance on foot.
Who’s right?
A Passing Wave
A puzzle from J.A.H. Hunter’s Fun With Figures (1956):
A man paddling a canoe upstream sees a glove in the water as he passes under a bridge. Fifteen minutes later, he turns around and paddles downstream. He passes under the bridge and travels another mile before reaching the rock from which he started, which the glove is just passing. If he paddled at the same speed the whole time and lost no time in turning around, what is the speed of the current?
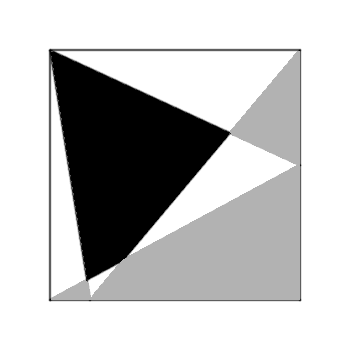
Modern Art
Black and White
Roll Call

A problem from the 2002 Moscow Mathematical Olympiad:
A group of recruits stand in a line facing their corporal. They are, unfortunately, rather poorly trained: At the command “Left turn!”, some of them turn left, some turn right, and some turn to face away from the corporal. Is it always possible for the corporal to insert himself in the line so that an equal number of recruits are facing him on his left and on his right?