Nine wolves share a square enclosure at the zoo. Build two more square enclosures to give each wolf a pen of its own.

Nine wolves share a square enclosure at the zoo. Build two more square enclosures to give each wolf a pen of its own.
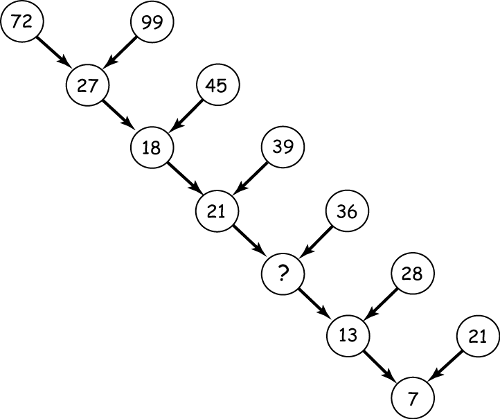
A conundrum by the late brilliant Japanese puzzle maven Nob Yoshigahara.
Lee Sallows writes, “You have to solve this yourself, otherwise you won’t see how beautiful it is.”
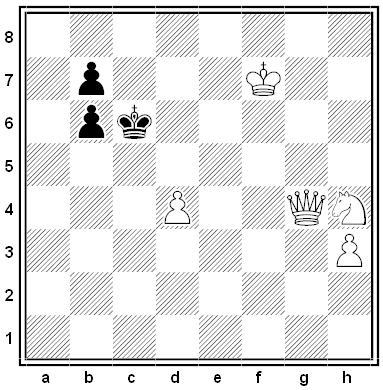
A cylindrical problem by Miodrag Petkovic: Imagine that the board is rolled into a cylinder, with the a-file adjoining the h-file. How can White mate in two moves?
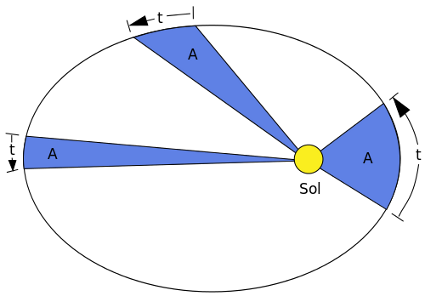
Kepler’s second law holds that a line segment connecting an orbiting planet to its sun sweeps out equal areas in equal periods of time: In the diagram above, if the time intervals t are equal, then so are the areas A.
If gravity were turned off, would this still be true?
Prove that log10 5 is irrational.
Imagine a 1000 x 1000 chessboard on which a white king and 499 black rooks are placed at random such that no rook threatens the king. And suppose the king goes bonkers and wants to kill himself. Can he reach a threatened square in a finite number of moves if Black is trying actively to avoid this?