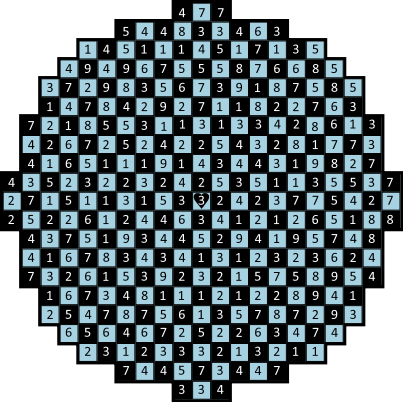
Sam Loyd devised this puzzle in 1898. Begin at the heart in the center and move three squares in any of the eight directions, north, south, east, west, northeast, northwest, southeast, or southwest. You’ll land on a number; take this as the length of your next “march,” which again can go in any of the eight directions. “Continue on in this manner until you come upon a square with a number which will carry you just one step beyond the border, thus solving the puzzle.”
Interestingly, Loyd devised this puzzle expressly to defeat Leonhard Euler’s method of solving mazes. “Euler, the great mathematician, discovered a rule for solving all manner of maze puzzles, which, as all good puzzlists know, depends chiefly upon working backwards. This puzzle, however, was built purposely to defeat Euler’s rule and out of many attempts is probably the only one which thwarts his method.” The original puzzle, as published in the New York Journal and Advertiser, contained a flaw that permitted multiple solutions. That’s been corrected here — there’s only one way out.
|
SelectClick for Answer |
Move:
- 3 squares southwest to 4.
- 4 squares southwest to 6.
- 6 squares northeast to 6.
- 6 squares northeast to 2.
- 2 squares northeast to 5.
- 5 squares southwest to 4.
- 4 squares southwest to 4.
- 4 squares southwest to 4.
- 4 squares southeast “to liberty.”
UPDATE: Wait, there’s at least one additional solution — in the last step you can go northwest as well as southeast. (Thanks, Carlos.) Now I wonder whether there’s ever been a version with a unique solution.
|